About Pythagorean Triples Formula
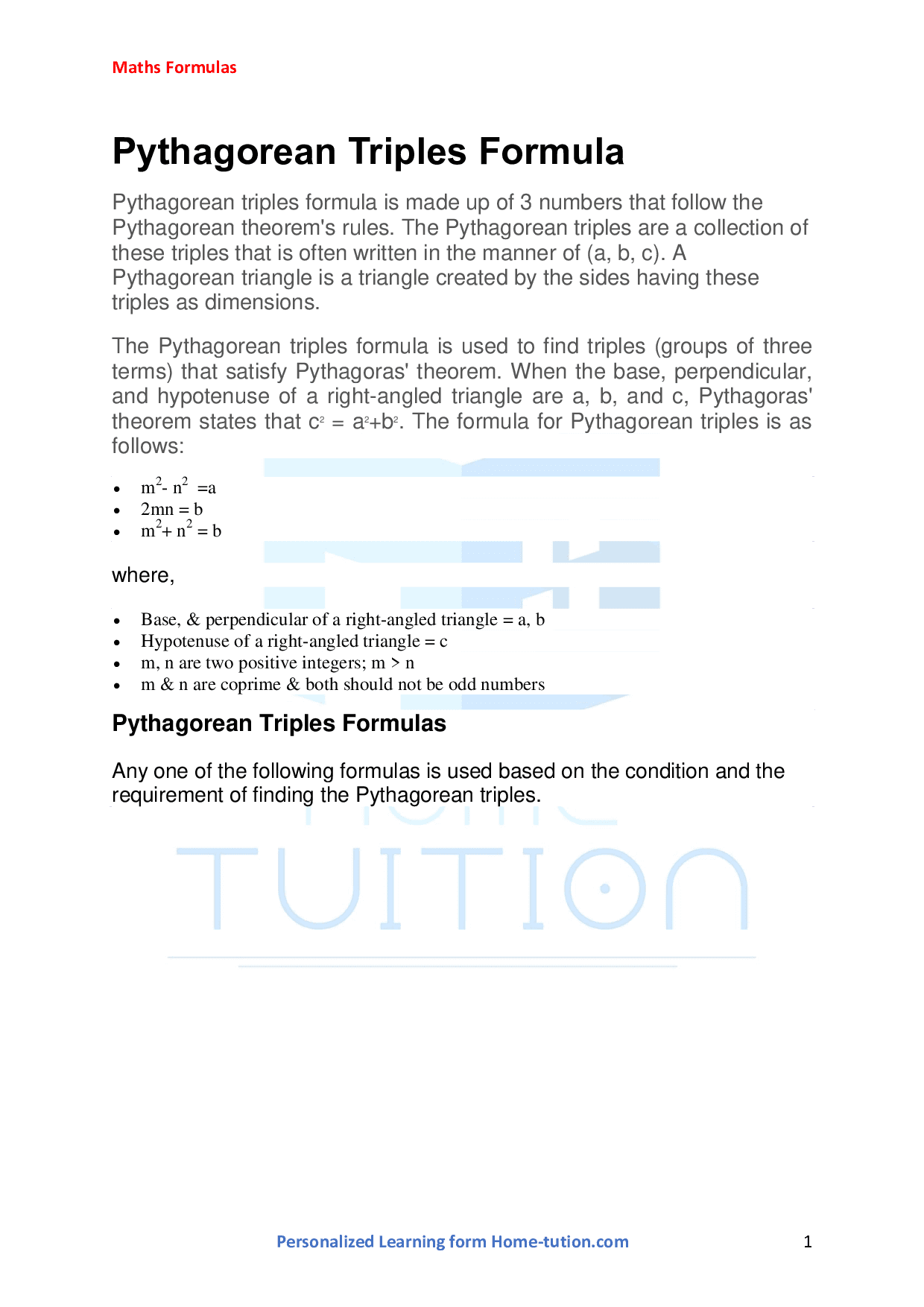
The Pythagorean triples formula is made up of 3 numbers that follow the Pythagorean theorem's rules. The Pythagorean triples are a collection of these triples that are often written in the manner of (a, b, c). A Pythagorean triangle is a triangle created by the sides having these triples as dimensions.
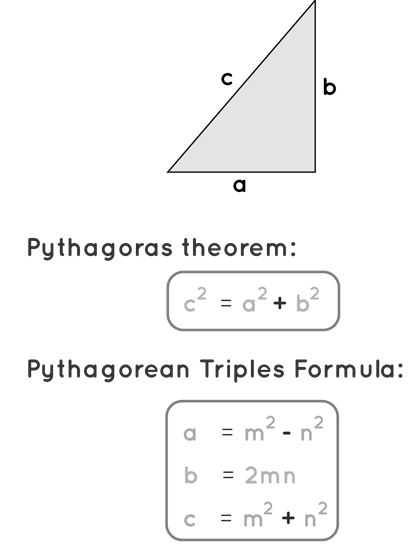
The Pythagorean triples formula is used to find triples (groups of three terms) that satisfy Pythagoras' theorem. When the base, perpendicular, and hypotenuse of a right-angled triangle are a, b, and c, Pythagoras' theorem states that c2 = a2+b2. The formula for Pythagorean triples is as follows: More Maths Formulas on the parent's page.
- m2- n2 =a
- 2mn = b
- m2+ n2 = b
where,
- Base, & perpendicular of a right-angled triangle = a, b
- Hypotenuse of a right-angled triangle = c
- m, n are two positive integers; m > n
- m & n are coprime & both should not be odd numbers
Pythagorean Triples Formulas
Any one of the following formulas is used based on the condition and the requirement of finding the Pythagorean triples.
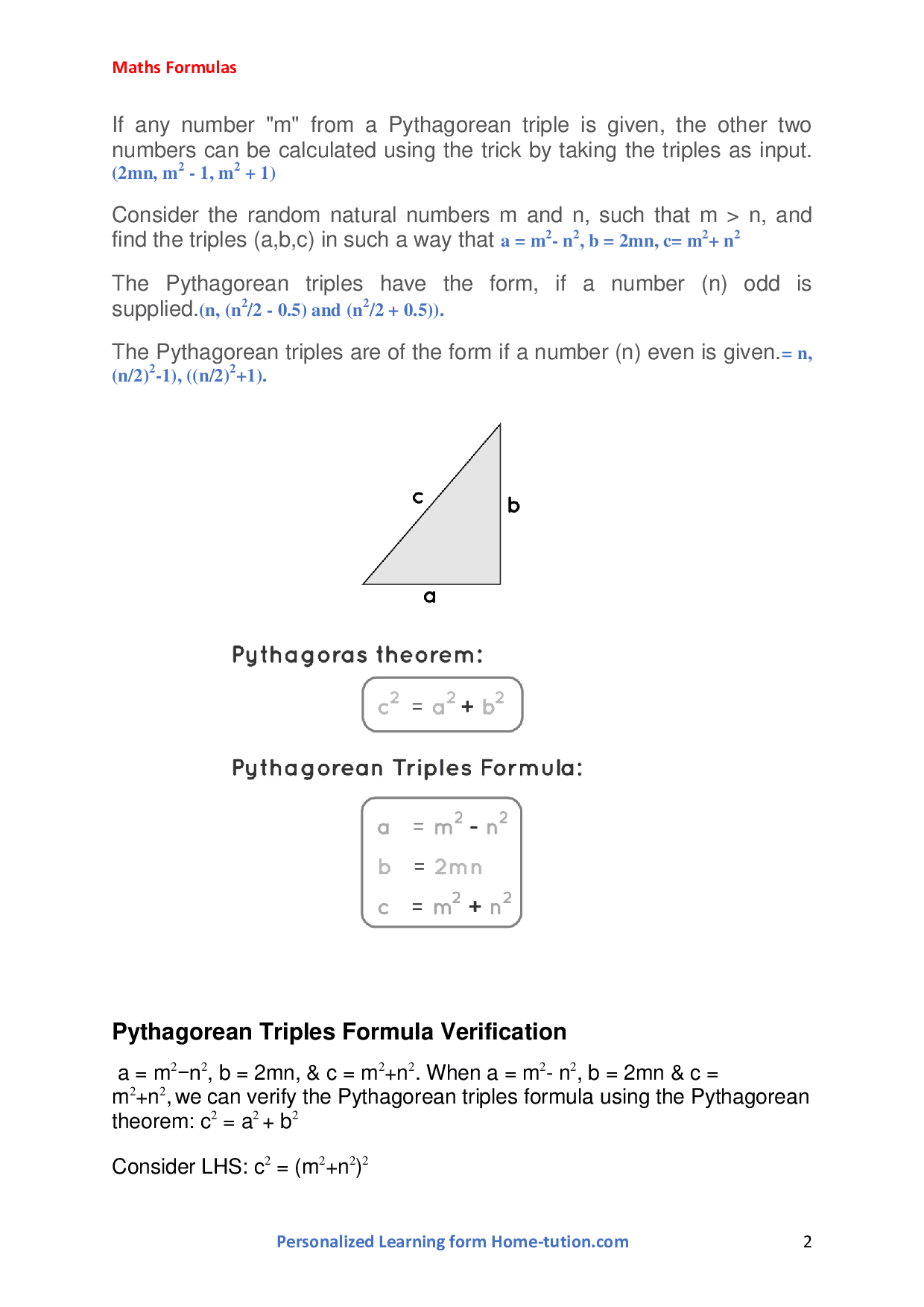
If any number "m" from a Pythagorean triple is given, the other two numbers can be calculated using the trick by taking the triples as input. (2mn,m2- 1, m2+ 1)
Consider the random natural numbers m and n, such that m > n, and find the triples (a,b,c) in such a way that a = m2- n2, b = 2mn, c=m2+ n2
The Pythagorean triples have the form, if a number (n) odd is supplied.(n, (n2/2 - 0.5) and (n2/2 + 0.5)).
The Pythagorean triples are of the form if a number (n) even is given.= n, (n/2)2-1), ((n/2)2+1).
Pythagorean Triples Formula Verification
a = m2−n2,b = 2mn,&c = m2+n2.When a = m2- n2, b = 2mn & c = m2+n2,we can verify the Pythagorean triples formula using the Pythagorean theorem:c2= a2+ b2
Consider LHS: c2= (m2+n2)2
c2=m4+n4+2m2n2
Consider RHS: a2+b2=(m2- n2)2+(2mn)2
=m4+n4-2m2n2+4m2n2
a2+b2=m4+n4+2m2n2
LHS= RHS
Thus c2= a2+ b2
Find the pdf of the Pythagorean Triples Formula???????
