About Area under the curve formula
The area of a curve in a given graph can be calculated using the Definite Integral. It has boundaries, or start and endpoints, within which the area under a curve is determined. To calculate the area of the curve f(x) with respect to the x-axis, use the limit points [a, b]. The related definite integral expression is ∫ba f(x)dx.
The total of the areas is integration, and definite integrals are used to calculate the area within limitations.
The use of integration to find the area of circles, parabolas, and ellipses dates back to the third century BC. Let's get a better understanding of definite integrals and their properties.
What is a definite integral?
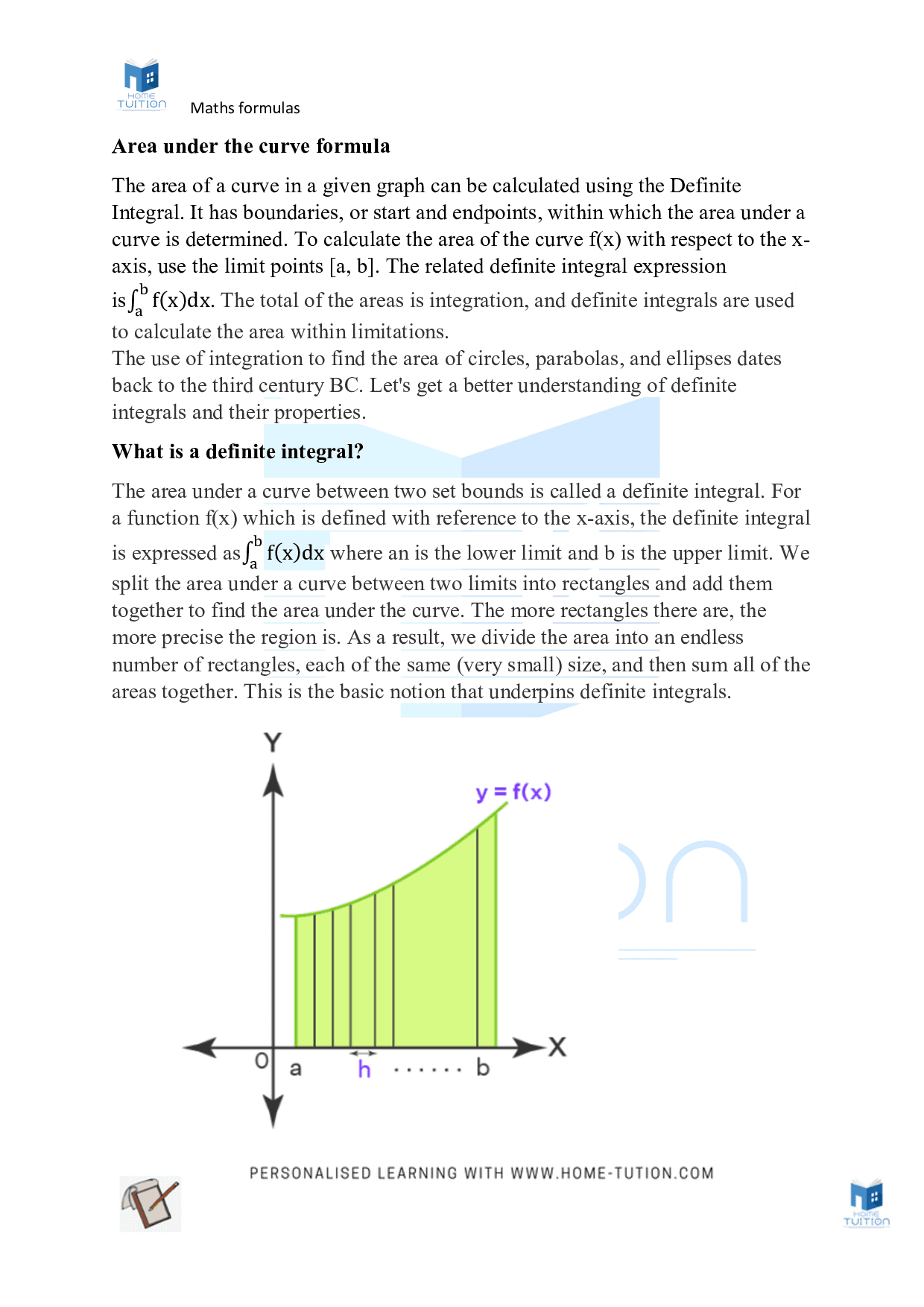
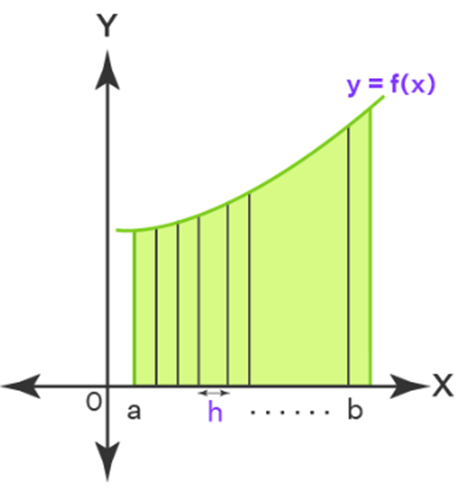
The area under a curve between two set bounds is called a definite integral. For a function f(x) which is defined with reference to the x-axis, the definite integral is expressed as ∫ba f(x)dx.where an is the lower limit and b is the upper limit. We split the area under a curve between two limits into rectangles and add them together to find the area under the curve. The more rectangles there are, the more precise the region is. As a result, we divide the area into an endless number of rectangles, each of the same (very small) size, and then sum all of the areas together. This is the basic notion that underpins definite integrals.
∫ba f(x)dx = F(b)−F(a), where F'(x) = f(x)
This can be evaluated by using the fundamental theorem of calculus (FTC).
To get all the Maths formulas check out the main page.
Find a free pdf for Area under the curve
