Area of a sector of a circle formula

About Area of a sector of a circle formula

Circle:

A circle is a geometrical object that is made up of an endless number of points in a plane that are spaced at a fixed distance from the circle's centre. The radius of the circle is the set distance between any of these locations and the centre

Area of a sector of a circle formula1

Arc:

A curve that runs around the perimeter of a circle.

Area of a sector of a circle formula2

The length of a sector's arc is calculated as follows: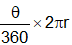Area of a sector of a circle formula3

 

It's possible that you won't be told the sector's angle. The arc's length is known instead. In certain cases, the formula below can be utilised to calculate the area.

Sector:

A sector is a segment of a circle defined by the arc connecting its two radii. A semi-circle, which represents half of a circle, is the most common sector of a circle.

A sector can be classified into two categories: Major Sectors and Minor Sectors.

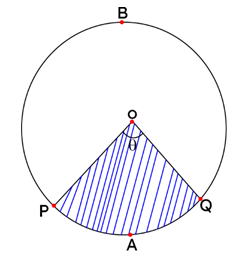
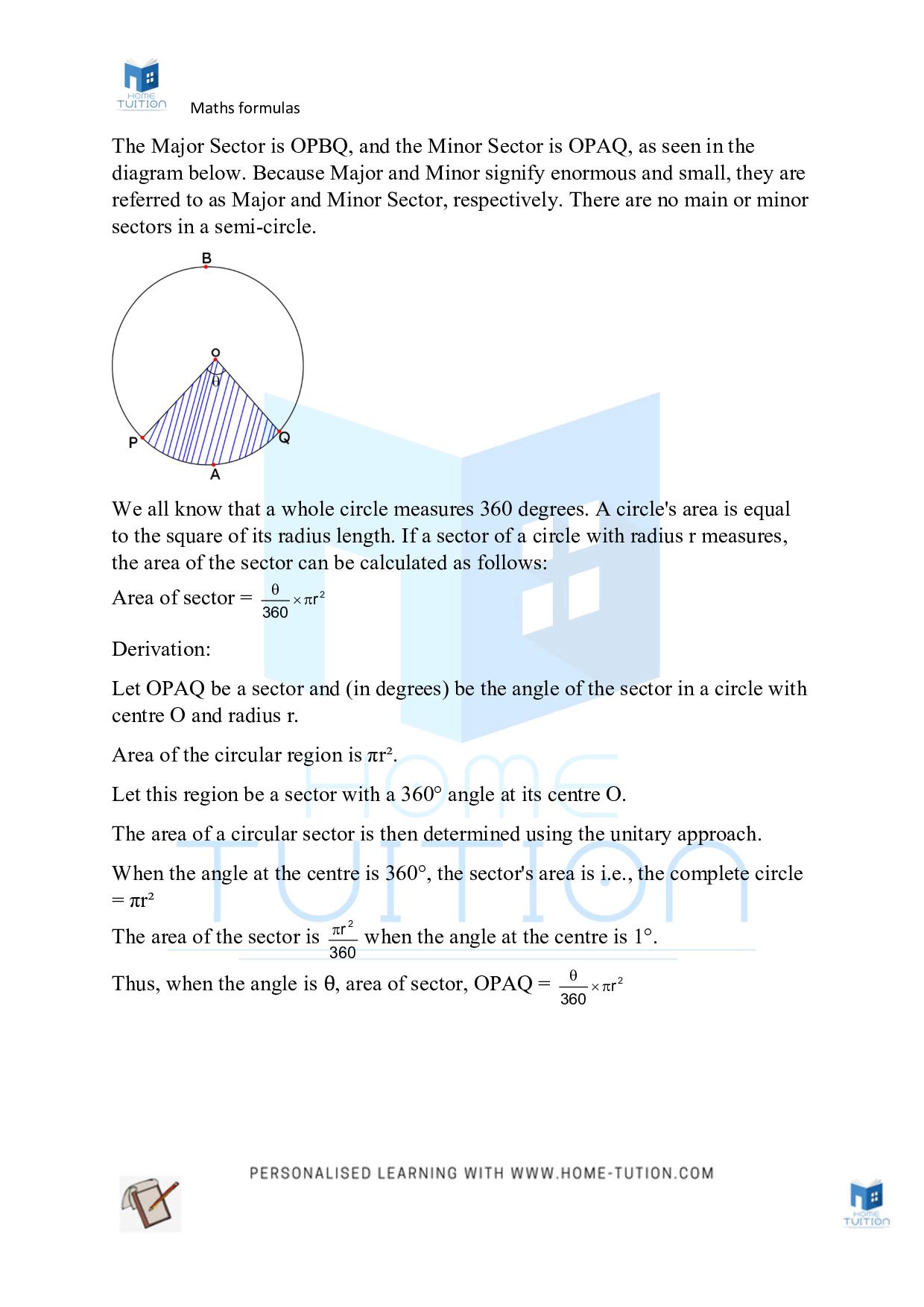
The Major Sector is OPBQ, and the Minor Sector is OPAQ, as seen in the diagram below. Because Major and Minor signify enormous and small, they are referred to as Major and Minor Sector, respectively. There are no main or minor sectors in a semi-circle.

Area of a sector of a circle formula4

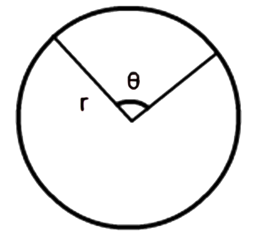
We all know that a whole circle measures 360 degrees. A circle's area is equal to the square of its radius length. If a sector of a circle with radius r measures, the area of the sector can be calculated as follows:

Area of sector = θ/360 × πr2

Derivation of Area of a sector of a circle formula

Let OPAQ be a sector and (in degrees) be the angle of the sector in a circle with centre O and radius r. Area of the circular region is πr2.

Let this region be a sector with a 360° angle at its centre O.

The area of a circular sector is then determined using the unitary approach.

When the angle at the centre is 360°, the sector's area is i.e., the complete circle = πr2

The area of the sector is when the angle at the centre is 1°.

Thus, when the angle is θ, the area of the sector, OPAQ = θ/360 × πr2

???????To get all the Maths formulas check out the main page. 

Find a Pdf of the area of a sector of a circle formula

Area of a sector of a circle formula
Area of a sector of a circle formula

Related Links

Frequently Asked Questions on Area of a sector of a circle formula