About Rational Numbers Formula
Let us review what rational numbers are before studying rational numbers formulas. A rational number is a fraction with an integer numerator and a non-zero integer denominator. However, not all fractions are rational numbers, as the numerator and/or denominator of a fraction might be irrational (s). In the next section, we'll go through the rational numbers formulas in depth. 'Q' stands for a set of rational numbers that includes:
- set of natural numbers - N
- set of whole numbers - W
- set of integers- W
- fractions of integers where the denominator is not zero.
Using the definition of a rational number from the previous section, a rational number is of the type pqpq, where p and q are integers and q0 is zero. Examples of rational numbers are 2, -1, -3/2, 1/3, 0, and so on. We manipulate rational numbers in the same manner that we manipulate fractions. The rational numbers formulas are as follows:
- Q={p/q : p,q∈Z;q≠0}
- x/y±m/n=xn±ym/yn
- x/y×m/n=xm/yn
- x/y÷m/n=xn/ym
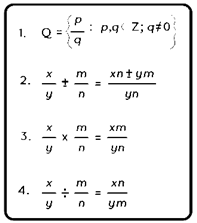
Note: Under addition and multiplication, the set of rational numbers is closed, associative, and commutative. The set of the rational numbers contains the additive identity, 0, and the multiplicative identity, 1. In the set of rational numbers, there are additive inverses for all rational numbers. In the set of rational numbers, all rational numbers other than 0 have their multiplicative inverses.
Download free pdf of Rational Numbers Formula Its Use And Solved Examples
