About Antiderivative Formula
Fundamental rules for determining the antiderivatives of various functions. Antidifferentiation is the opposite of differentiation, as the term implied as antiderivative formula
The antiderivative rules in calculus are fundamental rules for determining the antiderivatives of various functions. Antidifferentiation is the opposite of differentiation, as the term implies. These antiderivative rules can be used to find the antiderivative of sum or difference of functions, product and quotient of functions, scalar multiple of a function and constant function, and composition of functions. Antiderivative rules assist us in making the process of locating antiderivatives easier and more straightforward.
We'll go through the antiderivative rules in greater depth later in this article. For a better understanding of the topic, we will go over the antiderivative rules of various essential and specialised functions, as well as some solved cases.
What are Antiderivative Rules?
Antiderivative rules are some of the most significant principles for determining the antiderivatives of various sorts of function combinations. These antiderivative rules can be used to find the antiderivatives of functions such as product, quotient, sum, difference, scalar multiple, and composition
List of Antiderivative Rules
The following is a list of the most used antiderivative rules for the product, quotient, sum, difference, and function composition:
- Rule of Antiderivative Power
- Rule of Antiderivative Chains
- Rule Against Derivative Products
- Rule of Antiderivative Quotient
- Scalar Multiple of Function Antiderivative Rule
- Sum and Difference of Functions Antiderivative Rule
- Rule of Antiderivative Power
Now, antiderivative of power of x is given as
∫xn dx = xn+1/(n + 1) + C, where n ≠ -1.
Antiderivative Chain Rule
Because we know that antidifferentiation is the inverse of differentiation, the derivative rules lead to antiderivative rules. The antiderivative chain rule, commonly known as the u-substitution method of antidifferentiation, is derived from the derivative chain rule. If the integral has a form u'(x) f(u(x)) dx, the antiderivative chain rule is applied.
Antiderivative Product Rule
The integration by parts method is also known as the antiderivative product rule. It is one of the most significant antiderivative rules, and it is used to establish the antidifferentiation of a product of functions. The antiderivative product rule's formula is f (x). g(x) dx = f (x) (f′(x) [g(x) dx)] g(x) dx) dx + C.
This method of integration is also known as the ILATE or LIATE method of integration, which stands for:
I - Inverse Trigonometric Function
L - Logarithmic Function
A - Algebraic Function
T - Trigonometric Function
E - Exponential Function
Antiderivative Rule for Scalar Multiple of Function
We can calculate the antiderivative of a scalar multiple of a function f(x) using the formula kf(x) dx = k f(x) dx.
Sum and Difference Antiderivative Rule
This is now one of the most straightforward antiderivative rules. When determining the antidifferentiation of the sum and difference of functions, we can use these formulas:
∫[f(x) + g(x)] dx = ∫f(x) dx + ∫g(x) dx
∫[f(x) - g(x)] dx = ∫f(x) dx - ∫g(x) dx
Antiderivative Rules for Trigonometric Functions
Sine, cosine, tangent, cotangent, secant, and cosecant are the six major trigonometric functions. Now we'll look at their antiderivative rules for these trigonometric functions:
∫sin x dx = -cos x + C
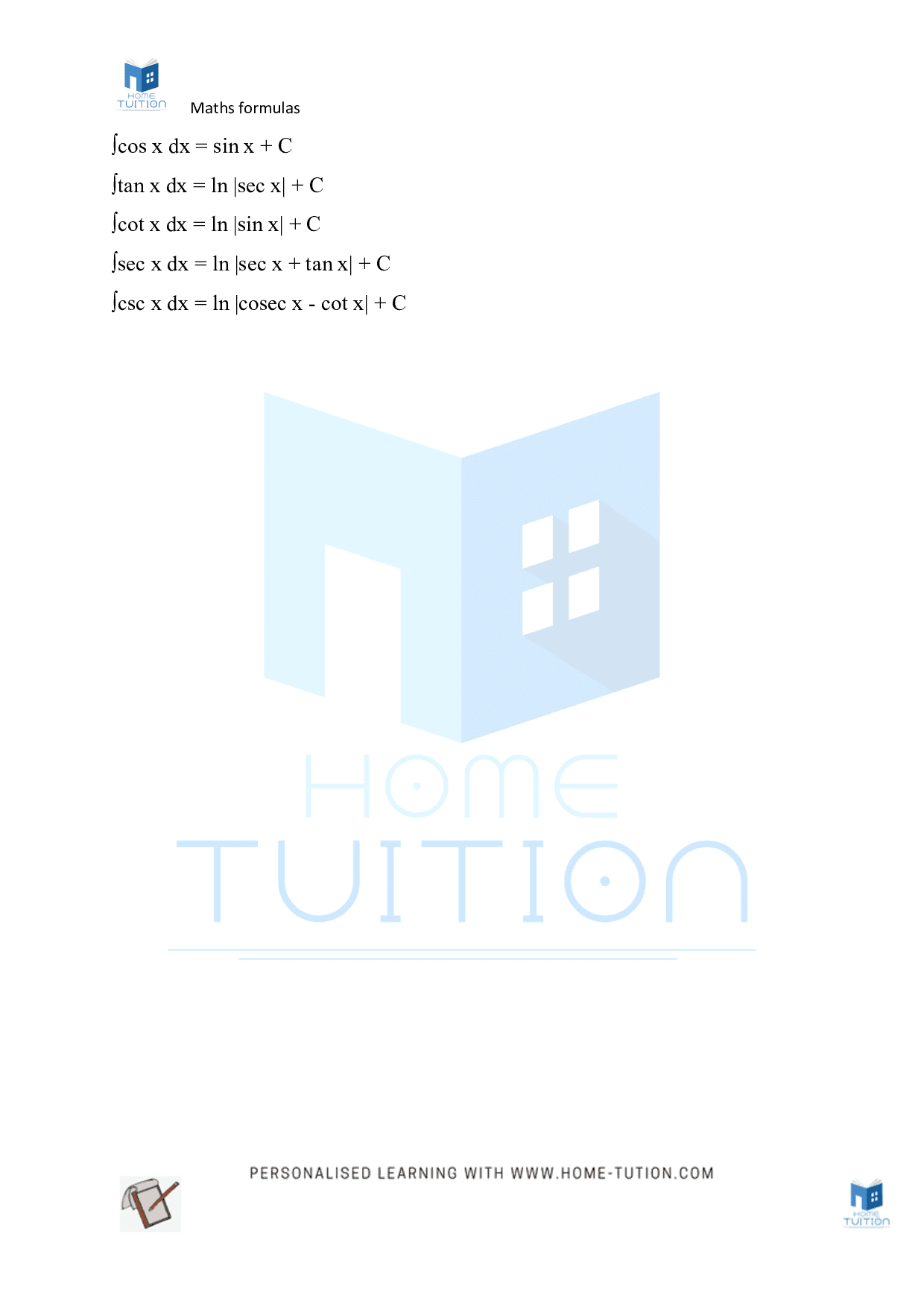
∫cos x dx = sin x + C
∫tan x dx = ln |sec x| + C
∫cot x dx = ln |sin x| + C
∫sec x dx = ln |sec x + tan x| + C
∫csc x dx = ln |cosec x - cot x| + C
To get all the Maths formulas check out the main page.