About Distribution Formula
A uniform distribution is a continuous probability distribution that describes occurrences that are equally likely to happen. Two parameters, a and b, constitute a uniform distribution, where an is the minimum value and b is the maximum value. It's usually written as u. (a, b). The probability density function is represented visually as a rectangle with a base of ba and a height of 1/(b-a). Get the List of all Maths formulas in one place.
What do you mean by Uniform Distribution Formula?
When f(x)=1/(b-a) is the probability density function or probability distribution of a uniform distribution with a continuous random variable X, it is represented by U(a,b), where a and b are constants such that a
f(x) = 1/ (b-a) for a≤ x ≤b.
Here, a is the minimum value, b is the maximum value
The probability density can be represented as follows in terms of mean μ and variance σ2:
f(x)={1/2σ√3 for −σ√3≤x−μ≤σ√3, f(x) = 0 otherwise
Uniform Distribution Formula:
F(x)=1/(b-a)
Mean =(a+b)/2
σ =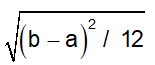
Example: The height of a uniform probability density function is as follows:
- Varies depending on the value of x
- Declines as x grow
- Is the same for every x value
Sol: A uniform probability distribution function is a symmetrical or uniformly distributed probability distribution of a finite continuous variable data series. Because it is a flat probability density, the area beneath it is equal to one. The median equals the mean, and all values are equally likely.
As a result, the height of a uniform probability density function is the same for all x values.
Answer: c is the same for each value of x
Find the pdf of Distribution Formula
