About Area of Trapezoid
The number of unit squares which can be fitted into a trapezoid's area is measured in square units (like cm2, m2, in2, etc). If a trapezoid can fit 15 unit squares of length 1 cm each inside it, then its area is 15 cm2. A trapezoid is a quadrilateral that has only one pair of parallel sides (which are known as bases). It implies that the second set of sides may not be parallel (which are known as legs). Drawing unit squares and measuring the area of a trapezoid is not always possible. So, on this page, we'll look at the formula for calculating the area of a trapezoid.
What is the Area of the Trapezoid?
The total space covered by the sides of a trapezoid is its area. It's worth noting that if we know the lengths of all the sides, we can simply divide the trapezoid into smaller polygons like triangles and rectangles, calculate their areas, and then add them up to get the trapezoid's area. However, if we know specific dimensions, we can apply a direct formula to find the area of a trapezoid.
Area of Trapezoid Formula
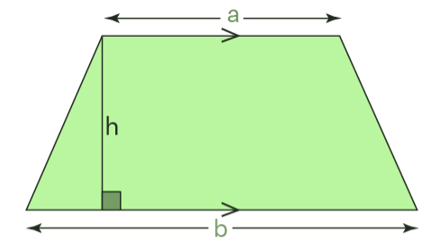
If the length of its parallel sides & the distance (height) between them are known, the area of a trapezoid can be computed. The area of a trapezoid is calculated using the formula,
A = ½ (a + b) h
where (A) is the trapezoid's area, a and b are its bases (parallel sides), and h is its height (the perpendicular distance between a and b)
A = ½ (a + b) h
Area of Trapezoid without Height
When all of the trapezoid's sides are known but the height is unknown, the area of the trapezoid can be calculated. In this scenario, we must first determine the trapezoid's height.
How to Derive Area of Trapezoid Formula?
The area of the trapezoid can be computed when all of the trapezoid's sides are known but the height is unknown. In this case, we must first determine the height of the trapezoid.
- Step 1: Cut a triangular section of the trapezoid as illustrated and split one of the legs into two equal parts.
- Step 2: Attach it to the bottom as shown, forming a large triangle.
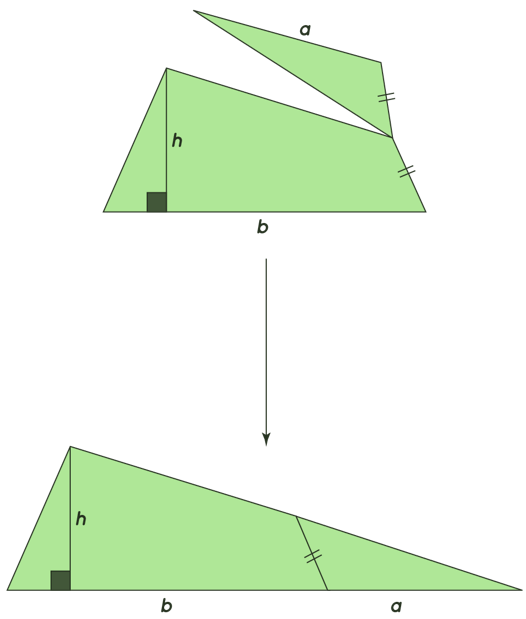
- Step 3: The trapezoid is reorganised into a triangle in this manner. We know that the area of the trapezoid and the new huge triangle remain the same when we attach it in this manner. We can also observe that the new huge triangle's base is (a + b) and that the triangle's height is h.
- Step 4: As a result, the area of the trapezoid equals the area of the triangle.
- Step 5: area of the trapezoid = × base × height = (a + b) h
We've now established the formula for calculating the area of a trapezoid.