About Hyperbola Formula
Hyperbolas are similar to mirrored parabolas in appearance. The branches are the two parts of the tree. A parabola is generated when the plane intersects the halves of a right circular cone, the angle of which is parallel to the cone's axis.
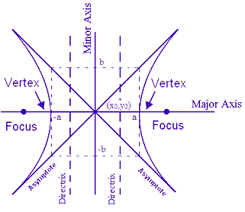
Two foci and two vertices make up a hyperbola. The hyperbola's foci are located away from the centre and vertices.
The equation for hyperbola is,(x - x0)2/a2 - (y - y0)/b2 = 1
Here, x0, y0 are the center points. a is semi-major axis.b is semi-minor axis.
MAJOR AXIS
The Major Axis is the line that runs through the centre, hyperbola's focus, and vertices. 2a is the length of the major axis.
The following is the equation: y = y0
MINOR AXIS
The Minor Axis is a line that runs orthogonal to the major axis and travels through the middle of the hyperbola. 2b is the length of the minor axis.
The following is the equation: x = x0
ECCENTRICITY
Eccentricity is the difference between the conic section being fully round and not. Hyperbola usually has a value larger than one. Eccentricity is a personality trait. 2√2 for a regular hyperbola.
Formula for eccentricity is: (√a2 + b2 /a)
ASYMPTOTES
The Asymptotes are two bisecting lines that pass through the centre of the hyperbola but do not touch the curve.
The following is the equation: (y = y0 + b/a x - b/a x0) ⇒ (y = y0 - b/a x + b/a x0)
Directrix of a hyperbola
A hyperbola's directrix is a straight line that is utilised to generate a curve. It is also known as the line away from which the hyperbola curves. This line runs perpendicular to the symmetry axis.
The directrix equation is: x = ± a2/√a2 + b2
VERTEX
The vertex is the point on a stretched branch that is closest to the centre. These are the vertex points.
(a,y0) and (- a,y0)
Focus (foci)
Focus (plural foci) are the fixed points on a hyperbola where the difference between the distances is always determined to be constant.
The following are the two focal points: (x0 + √a2 + b2, y0) and (x0 - √a2 + b2, y0)
Maths Formulas prepared by HT experts are listed on the main page.