Is this page helpful?
About Lagrange Interpolation Formula
Polynomial interpolation is done with Lagrange polynomials. For a particular set of distinct points and integers 
Lagrange’s interpolation is also an nth.
Approximation to f using degree polynomials (x).
Solved example based on Lagrange Interpolation Formula
Q-1 Find the formula for Lagrange Interpolation below.
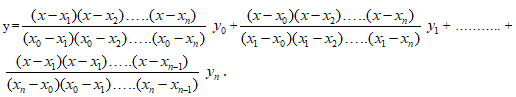
Example: Given a sequence of values (-2, 5), (1, 7), (3, 11), find the value of y at x = 0. (7, 34).
Sol: It is given that the known values are,
It is given that the known values are,
x = 0 ; x0 = -2 ; x1 = 1 ; x2 = 3 ; x3 = 7 ; y0 = 5 ; y1 = 7 ; y2 = 11 ; y3 = 34
Using the interpolation formula,
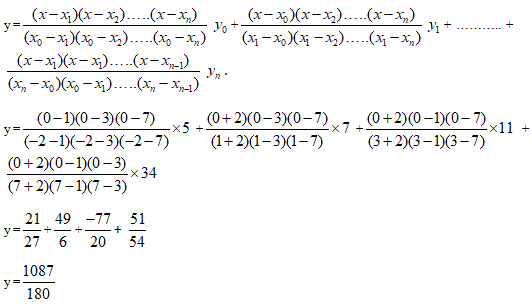
Download free pdf relative Error Formula Its Use And Solved Examples
