About The gradient of A Line
A line's gradient is defined as the change in its "y" coordinate about the change in its "x" coordinate. The gradient of a line can be used to determine its inclination or steepness. The slope of any line is calculated using the gradient of a line formula, which finds the ratio of the change in the y-axis to the change in the x-axis.
What Is Gradient of a Line?
The gradient of a line is the difference between its "y" and "x" coordinates. A line's inclination or steepness can be calculated using its gradient. The gradient of a line formula, which finds the ratio of change in the y-axis to change in the x-axis, is used to compute the slope of any line.
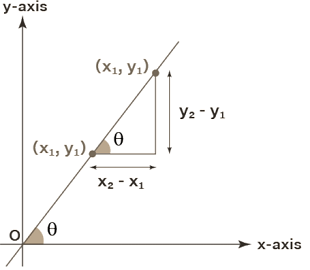
tan θ = (y2−y1)/(x2−x1)
m = tanθ
m = (y2−y1)/(x2−x1) = Δy/Δx, is the formula for calculating the gradient of a line. The gradient of the line is represented by m. The coordinates of the x-axis are x1x1, x2x2, while the coordinates of the y-axis are y1, y2. The gradient of the line, also known as the slope of the line, is calculated using the x and y coordinates of the point.
The gradient of a line is also represented as m = Δy/Δx which is the net change in the y coordinate with regard to the change in the x coordinate.
The change in y-coordinates is Δy , while the change in x-coordinates is Δx . We also know that tan is the slope of the line, where is the angle formed by the line with the positive axis direction, and tan=height/base. As a result, the line's gradient is m = tanθ = Δy/Δx
How To Find Gradient of a Line?
A line's gradient can be calculated using any two locations on the line.
- From Two Points: A line's gradient can be calculated using any two locations on the line. Regarding the two points, (x1,y1),(x2,y2), the gradient of the line is m = y2−y1 / x2−x1
- Angle Of Inclination: The line's inclination angle with respect to the x-axis is. The tangent of the angle of the inclination of the line w.r.t the x-axis yields the gradient of a line.
m = Tanθ. - Equation of a Line: A line's equation can also be used to determine its gradient. The gradient of the standard version of the equation of a line, which contains the equation ax + by + c = 0, is m = -a/b. And the gradient m is the coefficient of the x term in the slope-intercept form of the equation y = mx + c.
The gradient of a Line applications
In coordinate geometry, 3-dimensional geometry, and vector algebra, the gradient of a line has several uses. The following are some of the most important applications of a line's gradient.
- The inclination of a line concerning the x-axis is determined by its gradient.
- The equation of a line is found by using the gradient of a line.
- In three-dimensional geometry, the gradient of a line is used to find the equation of a line and the equation of a plane.
- The gradient of two lines can be used to determine the angle between them.
- The gradient of two lines can be used to determine whether they are parallel or perpendicular to one another.
- m1.m2 is equal to the product of the gradients of two perpendicular lines.
- The gradient of two parallel lines has the same value. m1=m2
Get the list of all Maths formulas used in general calculations.