NCERT Solutions for Chapter 7 Can You See The Pattern?
NCERT Solutions for Class 5 Maths Chapter 7 Can You See The Pattern? of the NCERT textbook is solved with errorless solutions by experts on Home-tuition.com. Chapter 7 Can You See The Pattern? is fully solved in the below pdf. You can download chapter-wise free pdf of chapter-Chapter 7 Can You See The Pattern?.
What are you going to learn in Chapter 7 Can You See The Pattern?
Can You See the Pattern?-Your skirt is stunning, Isha. This pattern was created by my mother. On a kurta, I've seen the same block used to create a different pattern. What made it unique? The rule of the pattern in your skirt is one up, one down. The process is then repeated. However, in my brother's kurta, it only goes up once and then takes a quarter turn every time. Repeat the process with a 1/4 clockwise spin. With the help of blocks, you can now create patterns using these rules. we will study different patterns.
Your skirt is stunning, Isha. This pattern was created by my mother. On a kurta, I've seen the same block used to create a different pattern. What made it unique? The rule of the pattern in your skirt is one up, one down. The process is then repeated. However, in my brother's kurta, it only goes up once and then takes a quarter turn every time. Repeat the process with a 1/4 clockwise spin. With the help of blocks, you can now create patterns using these rules. we will study different patterns.
Find below a free pdf of NCERT Solutions for Class 5 Maths Chapter 7 Can You See The Pattern?
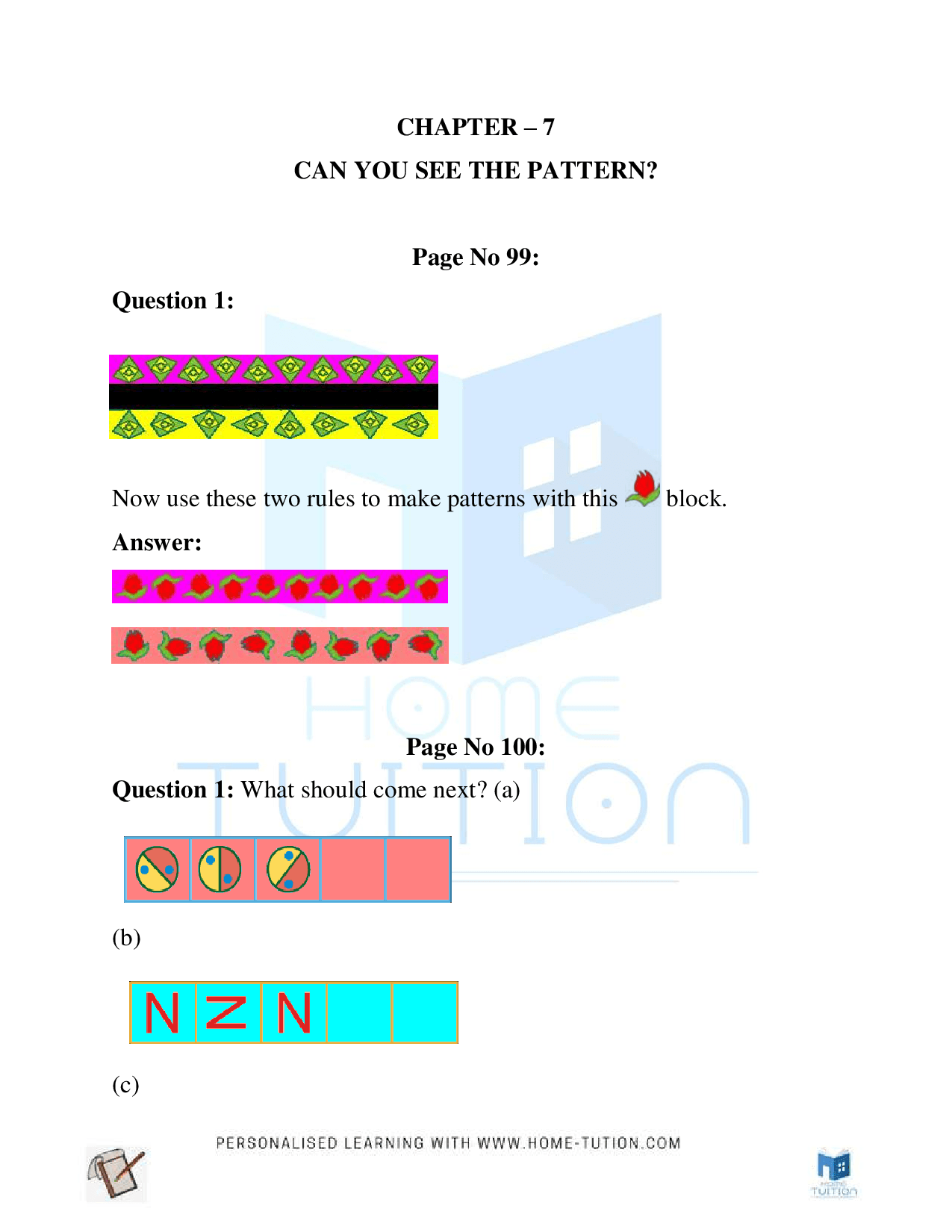
Page No 99:
Question 1:

Now use these two rules to make patterns with this ![]() block.
block.
Answer:
![]()

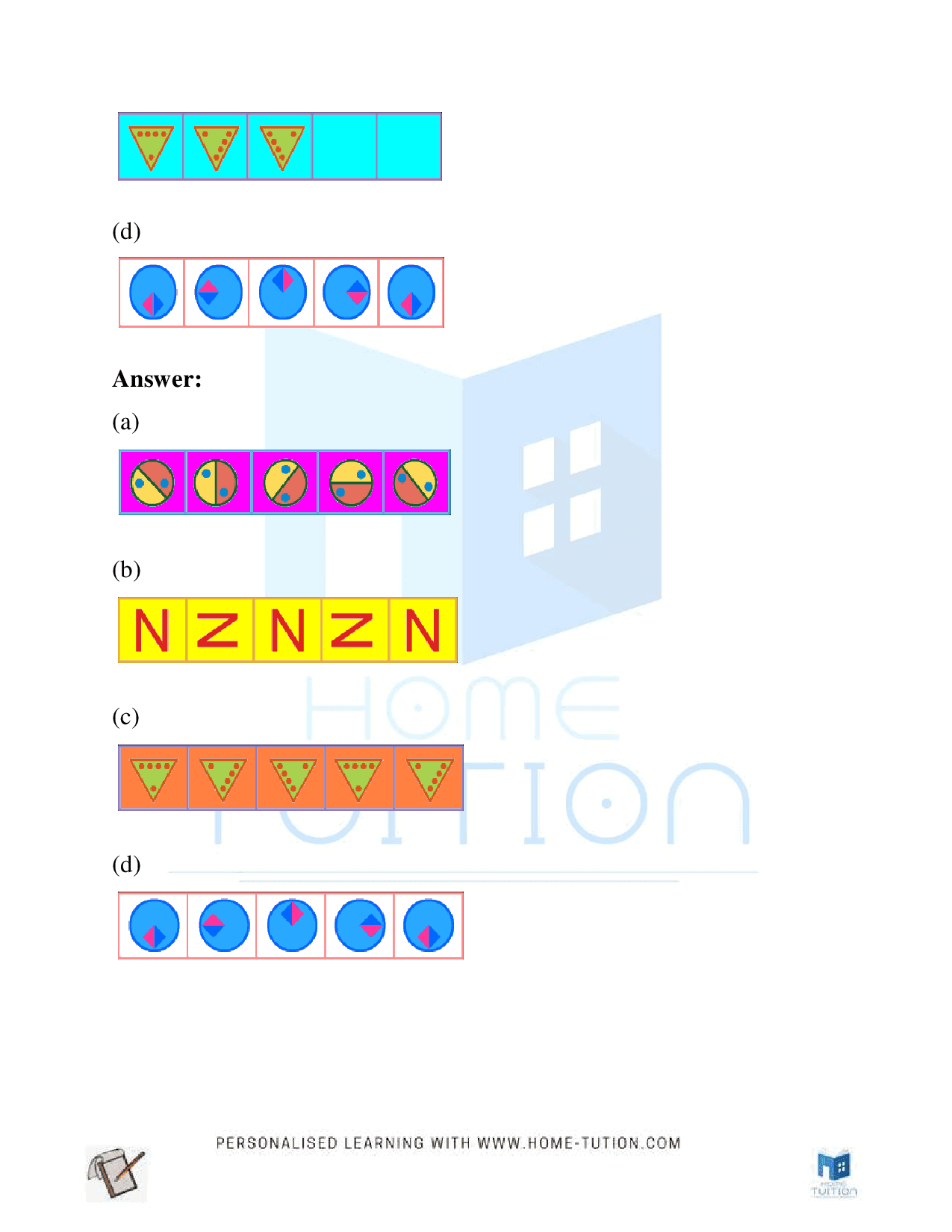
Page No 100: Question 1: What should come next?
(a)

(b)

(c)

(d)

Answer:
(a)

(b)

(c)

(d)

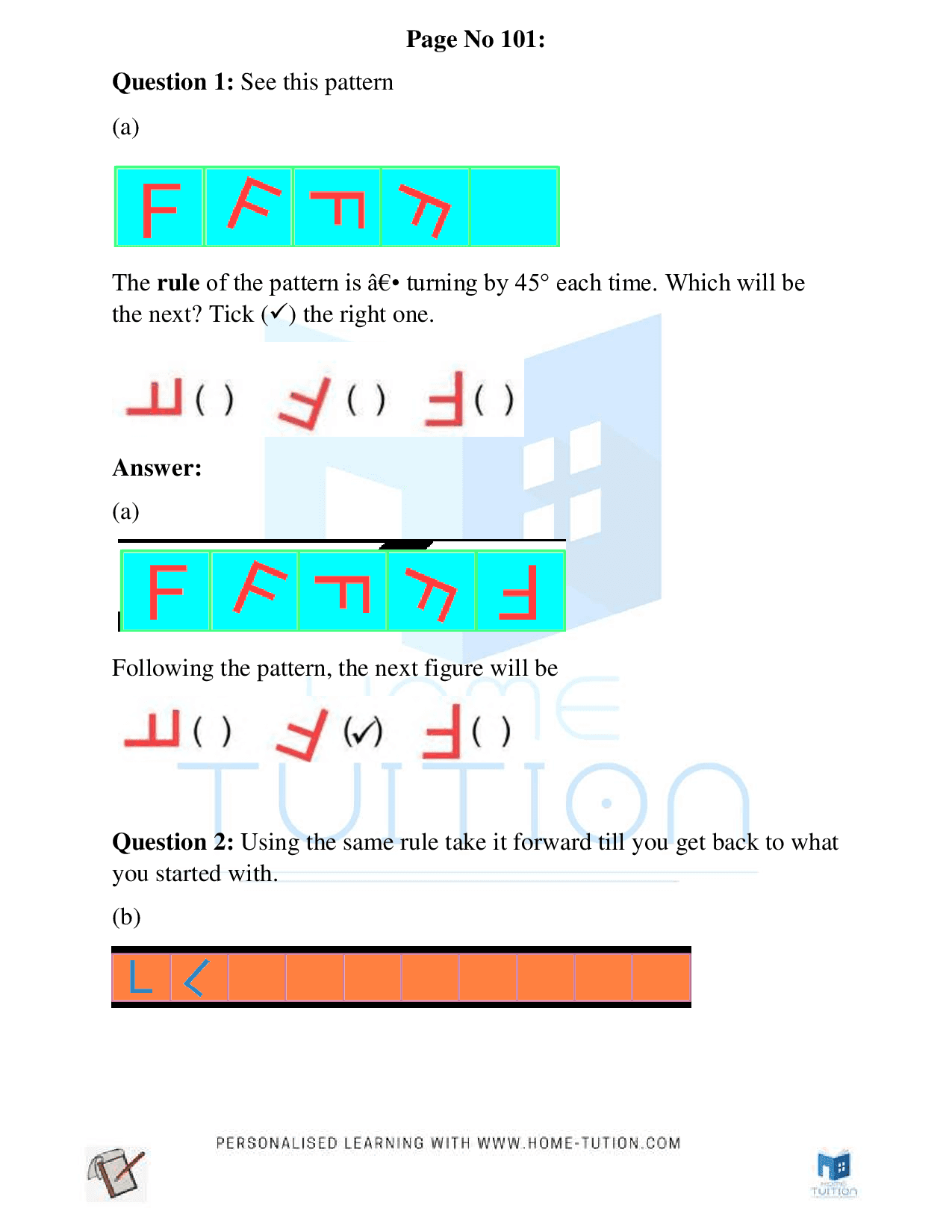
Page No 101:
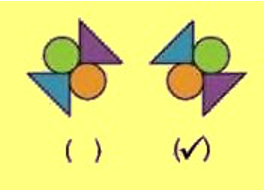
Question 1: See this pattern
(a)

The rule of the pattern is ― turning by 45° each time. Which will be the next? Tick () the right one.

Answer:
(a)

Following the pattern, the next figure will be

Question 2: Using the same rule take it forward till you get back to what you started with.
(b)

(c)

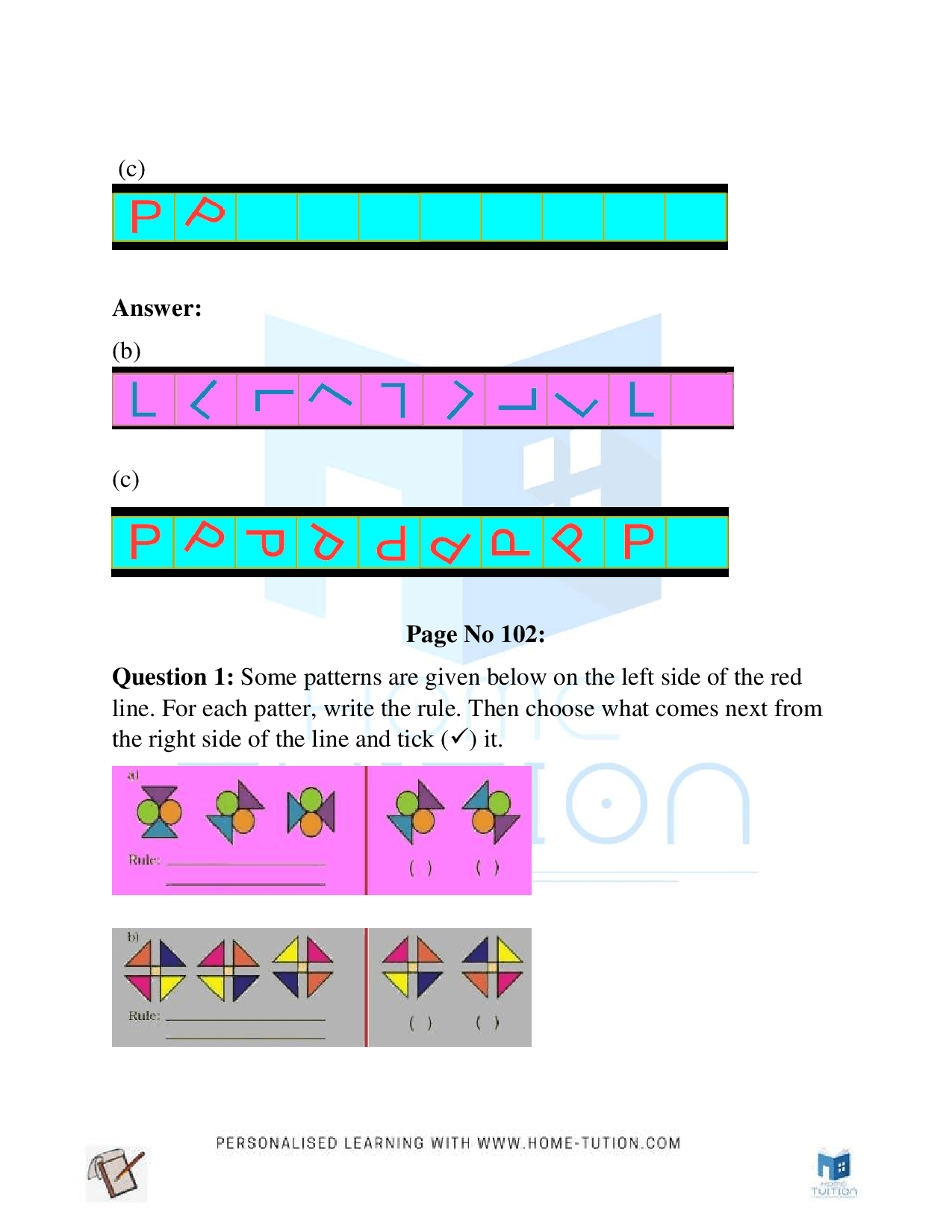
Answer:
(b)

(c)

Page No 102:
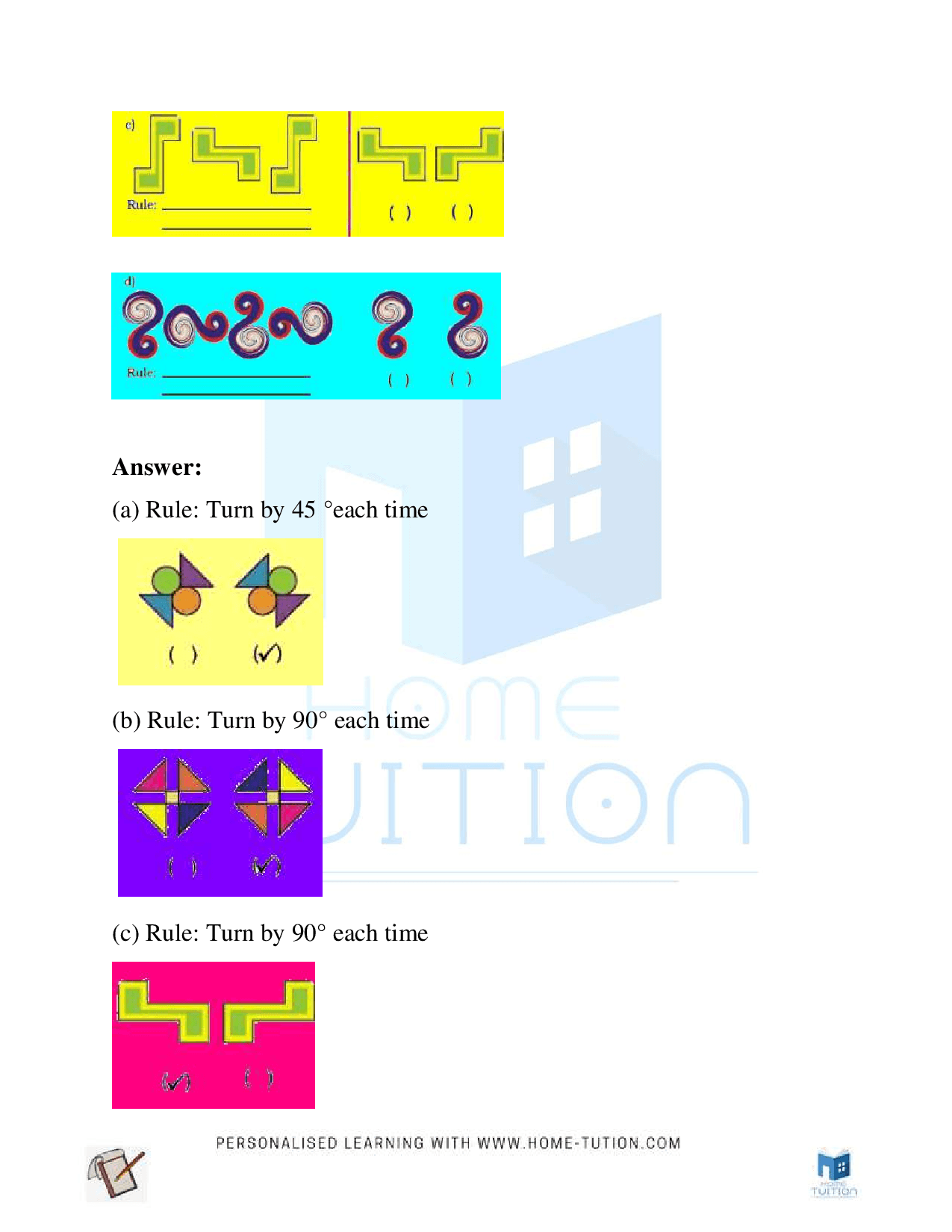
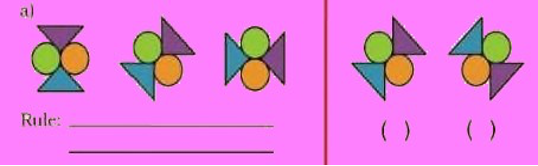
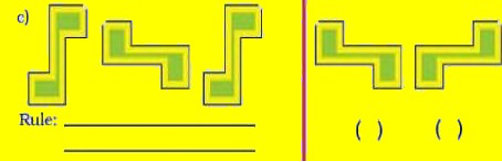
Question 1: Some patterns are given below on the left side of the red line. For each patter, write the rule. Then choose what comes next from the right side of the line and tick () it.


Answer:
(a) Rule: Turn by 450 each time
(b) Rule: Turn by 90° each time
(c) Rule: Turn by 90° each time
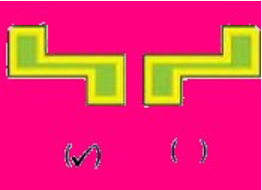
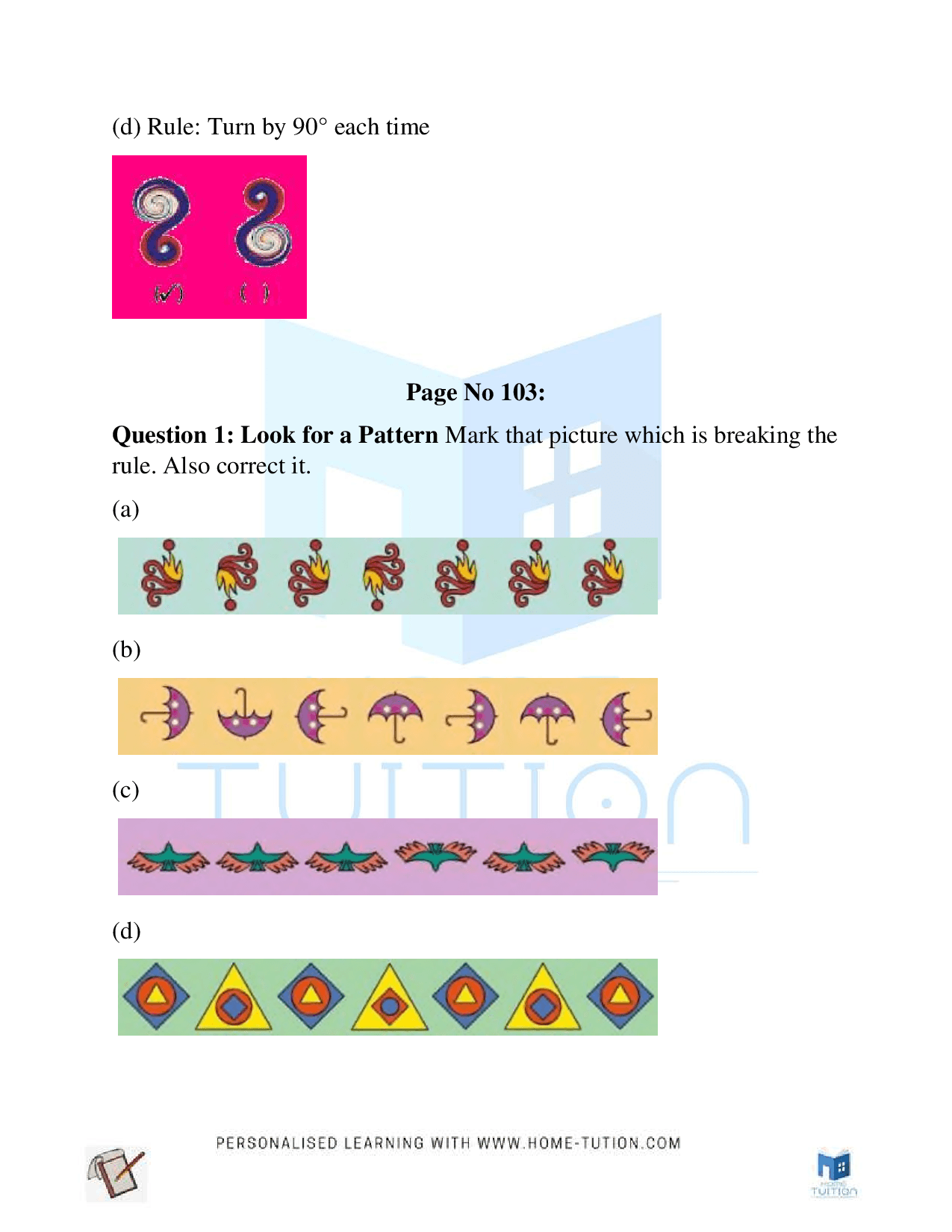
(d) Rule: Turn by 90° each time

Page No 103:
Question 1: Look for a Pattern Mark that picture which is breaking the rule. Also correct it.
(a)

(b)

(c)

(d)

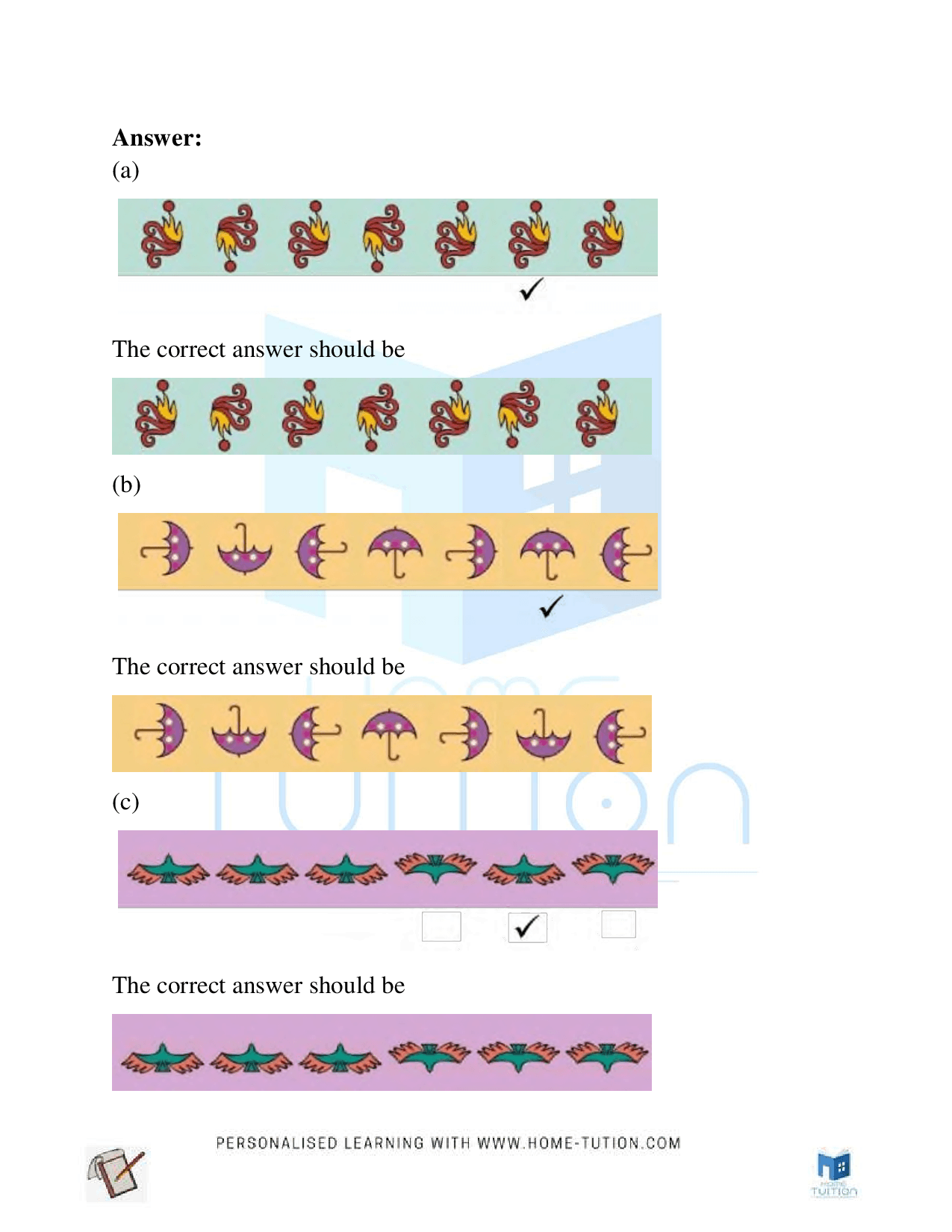
Answer:
(a)

The correct answer should be

(b)

The correct answer should be

(c)

The correct answer should be

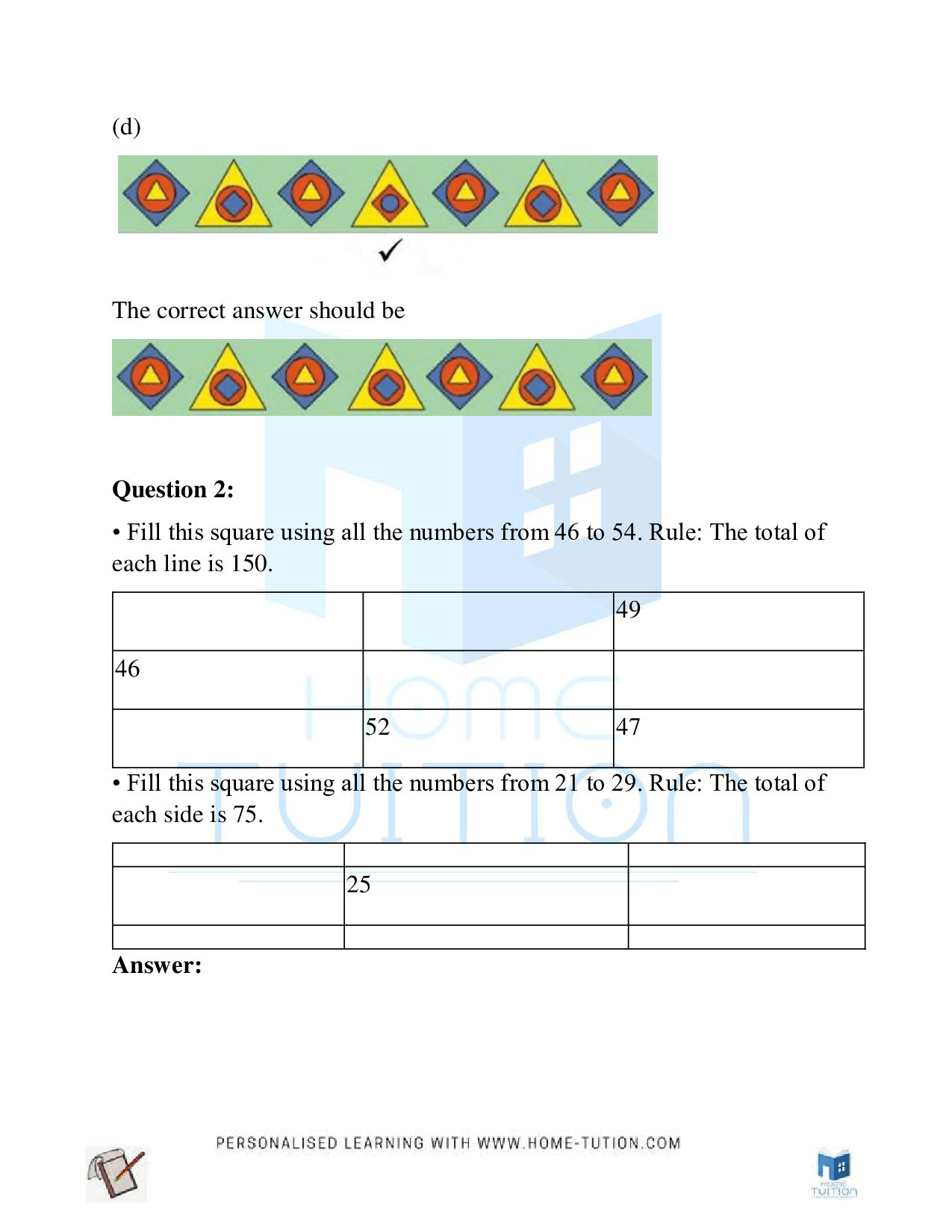
(d)

The correct answer should be
Question 2:
Fill this square using all the numbers from 46 to 54. Rule: The total of each line is 150.
|
49 |
||
|
46 |
||
|
52 |
47 |
Fill this square using all the numbers from 21 to 29. Rule: The total of each side is 75.
|
25 |
||
Answer:
|
53 |
48 |
49 |
|
46 |
50 |
54 |
|
51 |
52 |
47 |
|
24 |
23 |
28 |
|
29 |
25 |
21 |
|
22 |
27 |
26 |
Page No 104:
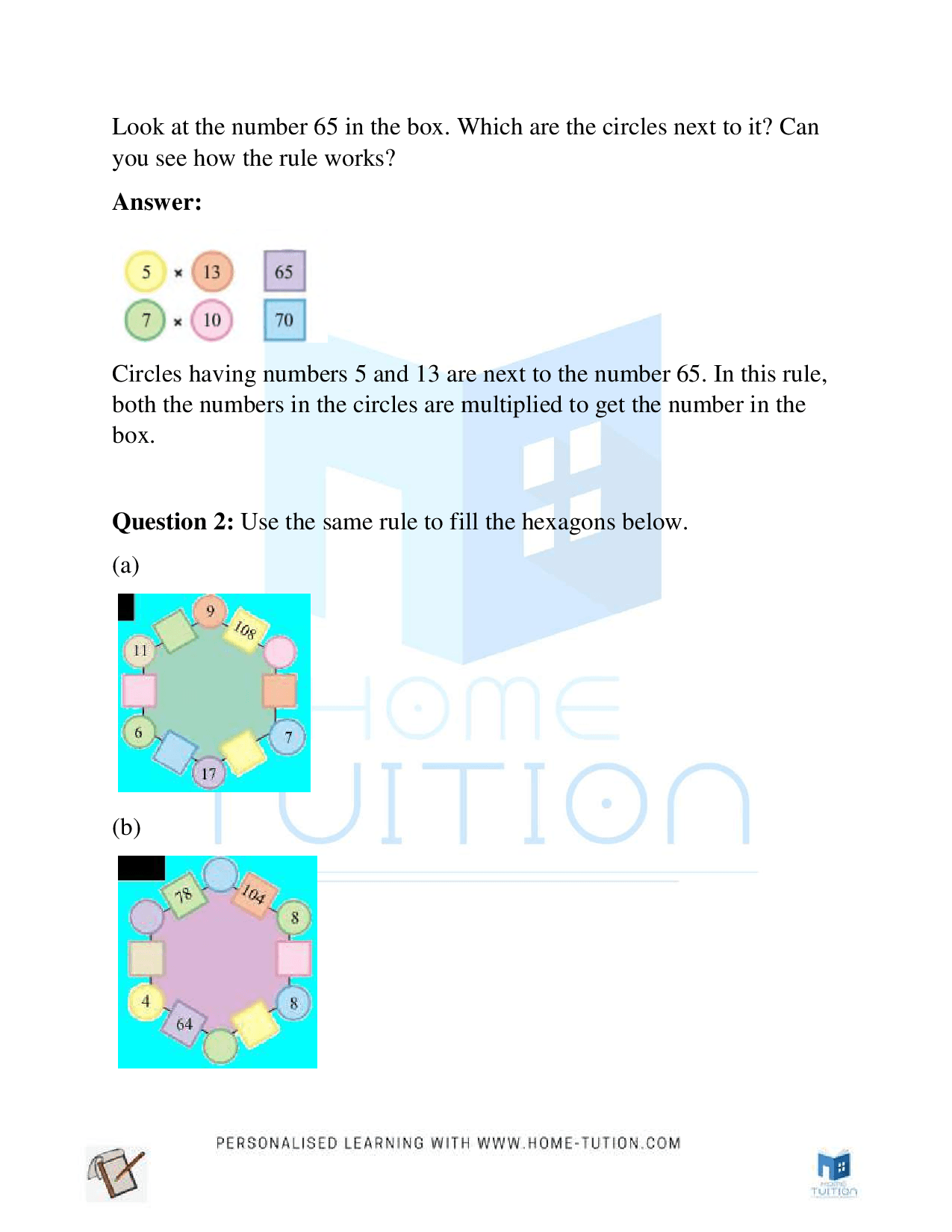
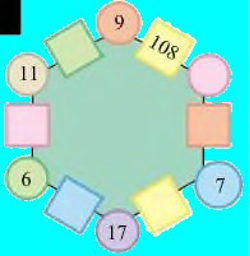
Question 1: Look at the patterns of numbers in hexagons. Each side has 2 circles and 1 box.
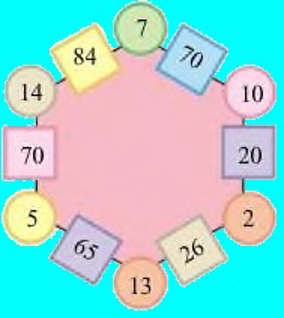

Look at the number 65 in the box. Which are the circles next to it? Can you see how the rule works?
Answer:
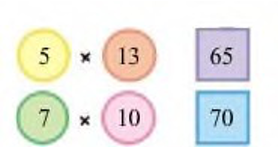
Circles having numbers 5 and 13 are next to the number 65. In this rule, both the numbers in the circles are multiplied to get the number in the box.
Question 2: Use the same rule to fill the hexagons below.
(a)
(b)

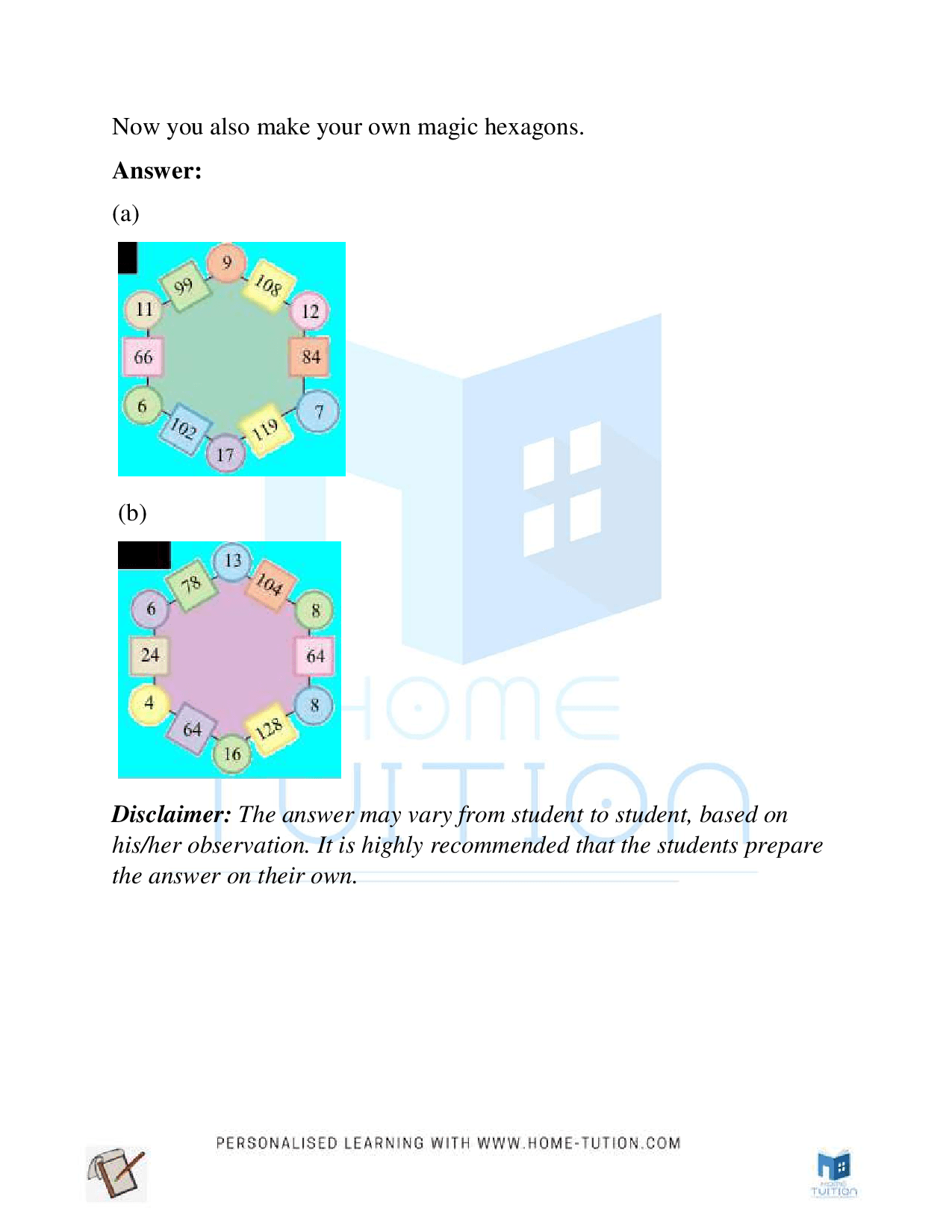
Now you also make your own magic hexagons.
Answer:
(a)
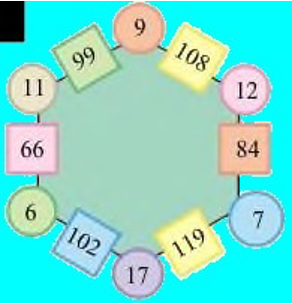
(b)

Disclaimer: The answer may vary from student to student, based on his/her observation. It is highly recommended that the students prepare the answer on their own.
Page No 105:
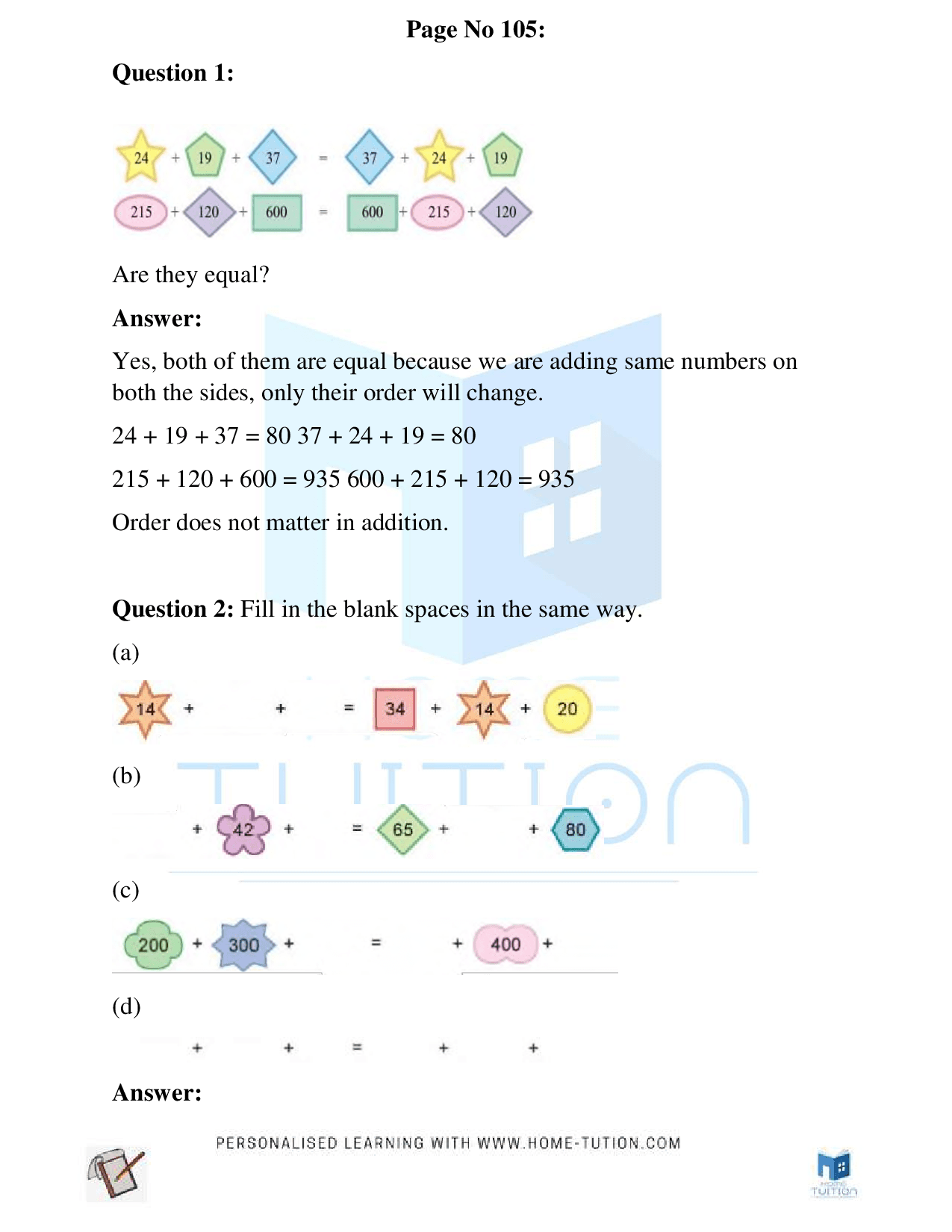
Question 1:
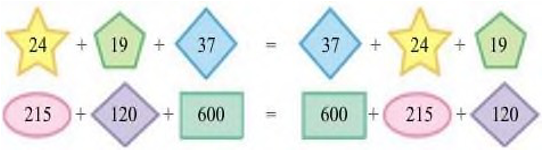
Are they equal?
Answer:
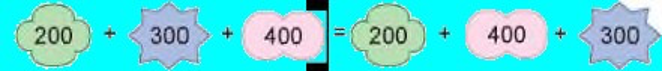
Yes, both of them are equal because we are adding same numbers on both the sides, only their order will change.
24 + 19 + 37 = 80 37 + 24 + 19 = 80
215 + 120 + 600 = 935 600 + 215 + 120 = 935
Order does not matter in addition.
Question 2: Fill in the blank spaces in the same way.
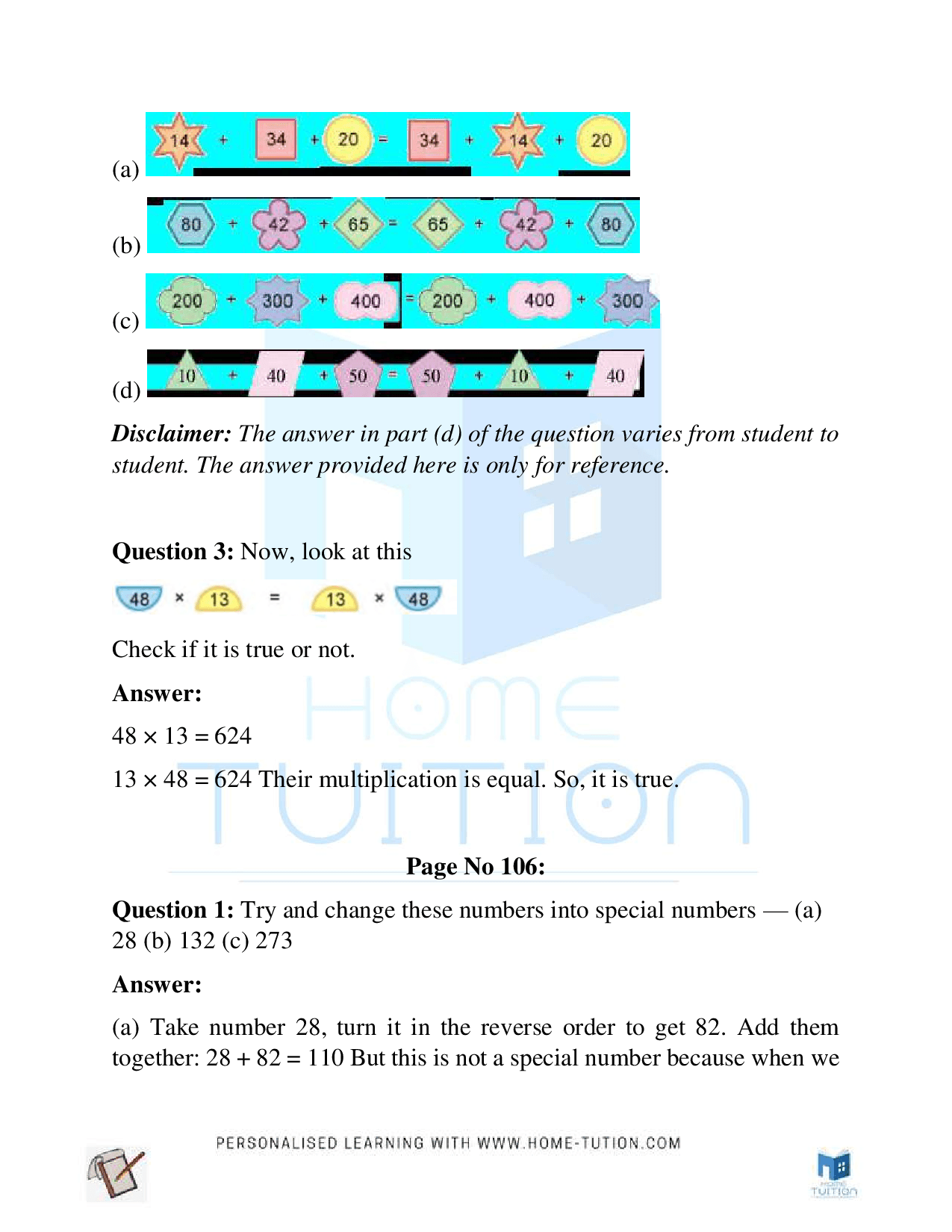
(a)
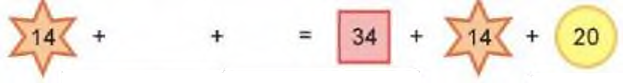
(b)

(c)
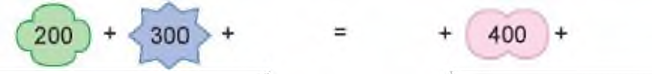
(d)
![]()
Answer:
(a)
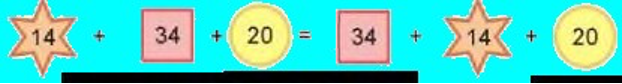
(b)

(c)
(d)

Disclaimer: The answer in part (d) of the question varies from student to student. The answer provided here is only for reference.
Question 3: Now, look at this
![]()
Check if it is true or not.
Answer:
48 × 13 = 624
13 × 48 = 624 Their multiplication is equal. So, it is true.
Page No 106:
Question 1: Try and change these numbers into special numbers
(a) 28
(b) 132
(c) 273
Answer:
(a) Take number 28, turn it in the reverse order to get 82. Add them together: 28 + 82 = 110 But this is not a special number because when we
reverse its order, we get 011. Now, we add: 110 + 011 = 121 When we reverse its order, we get 121. Thus, it is a special number.
(b) Take the number 132, turn it in the reverse order to get 231. Add them together: 132 + 231 = 363 When we reverse its order, we get 363. Thus, we get the special number as required.
(c) Take the number 273, turn it in the reverse order to get 372 Add them together: 273 + 372 = 645 But this is not a special number because when we reverse its order, we get 546. Now, if we subtract them, we get 645 − 546 = 99 When we reverse its order, we get 99, which is a special number.
Question 2: NO LEMONS NO MELON STEP NOT ON PETS
Did you notice that it reads the same from both sides — right to left and left to right? Now try and use words in a special way.
Answer:
MADAM, EYE, RACE CAR, A SANTA AT NASA
Disclaimer: The answer may vary from student to student. It is highly recommended that the students prepare the answer on their own. The answer provided here is only for reference.
Page No 107:
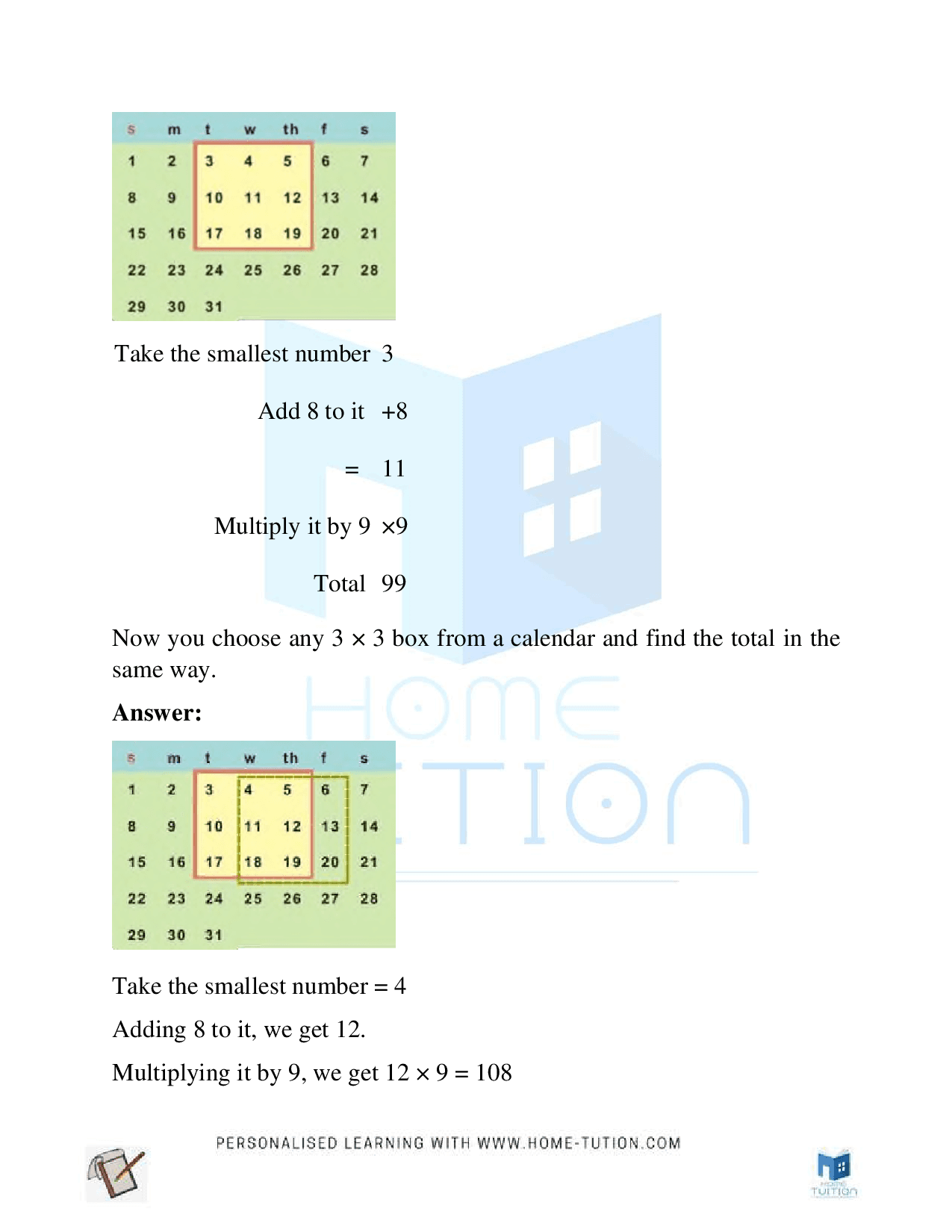
Question 1: Look at the calendar below. Let us mark a 3 × 3 box (9 dates) on the calendar and see some magic.
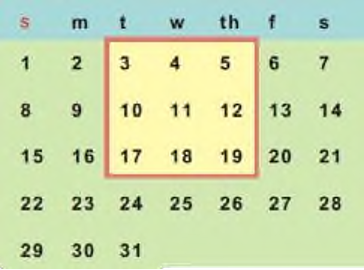
|
Take the smallest number |
3 |
|
Add 8 to it |
+8 |
|
= |
11 |
|
Multiply it by 9 |
×9 |
|
Total |
99 |
Now you choose any 3 × 3 box from a calendar and find the total in the same way.
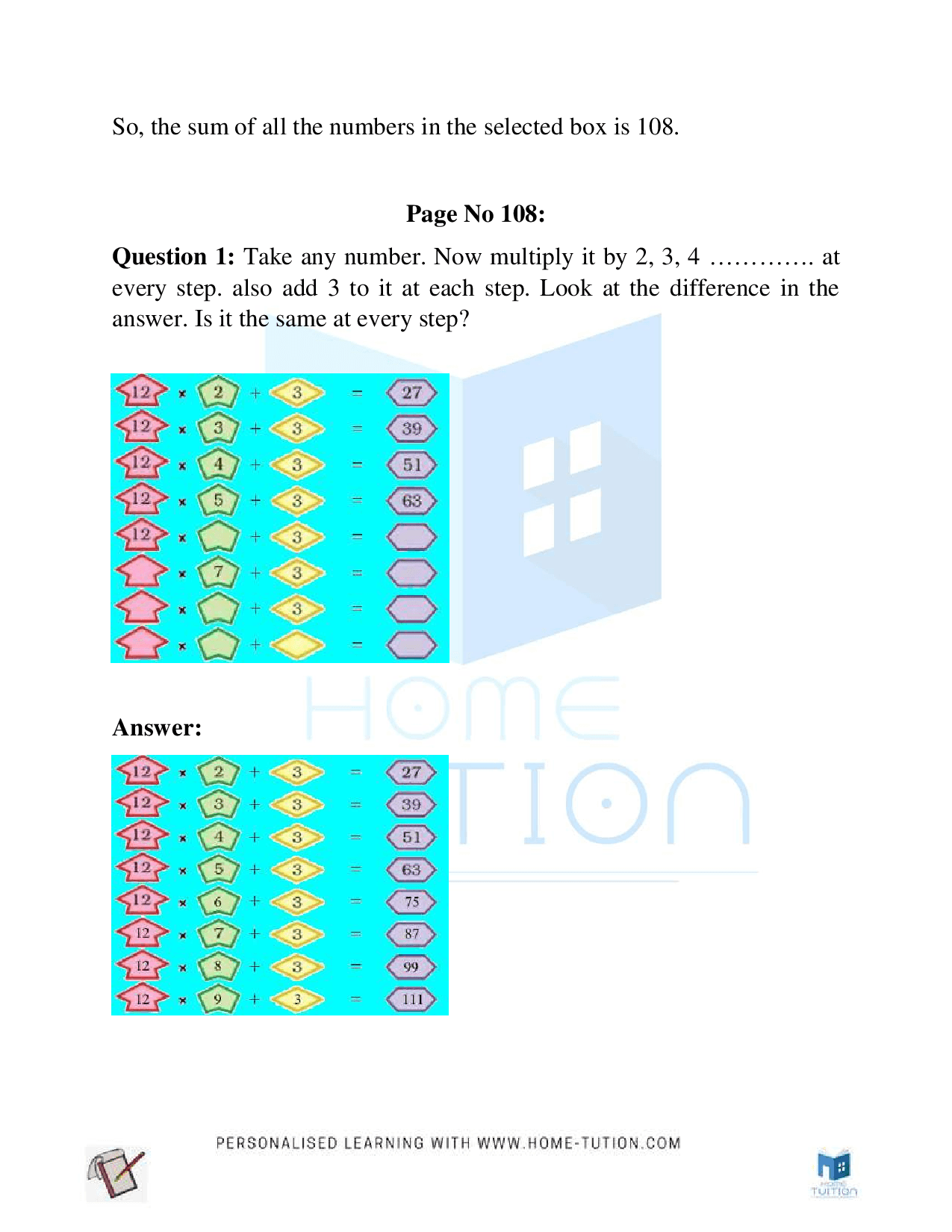
Answer:
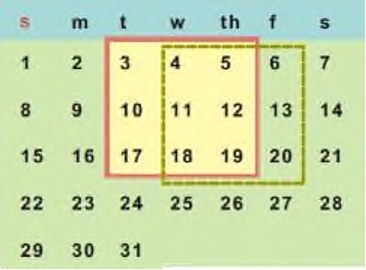
Take the smallest number = 4 Adding 8 to it, we get 12.
Multiplying it by 9, we get 12 × 9 = 108
So, the sum of all the numbers in the selected box is 108.
Page No 108:
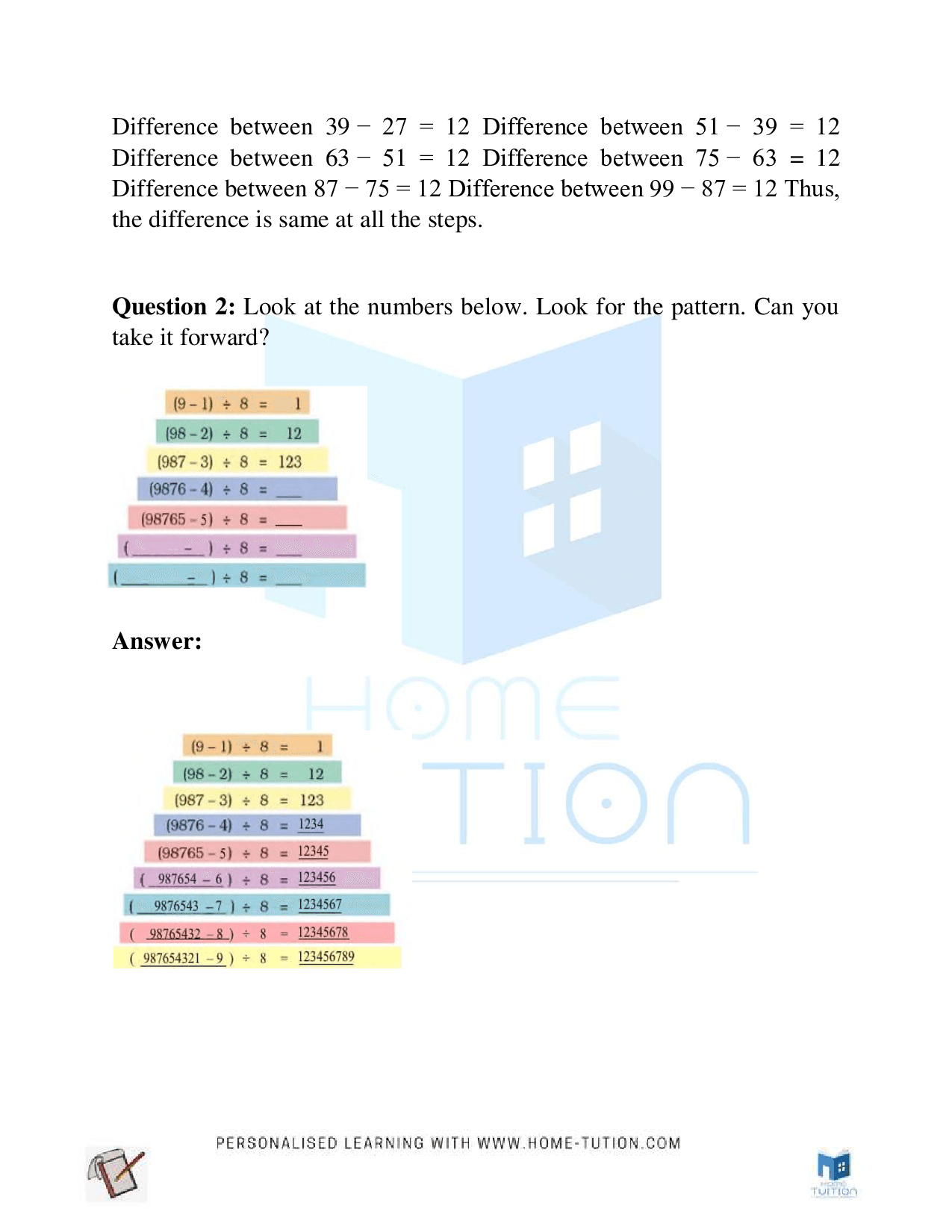
Question 1: Take any number. Now multiply it by 2, 3, 4 _____________at every step. also add 3 to it at each step. Look at the difference in the answer. Is it the same at every step?
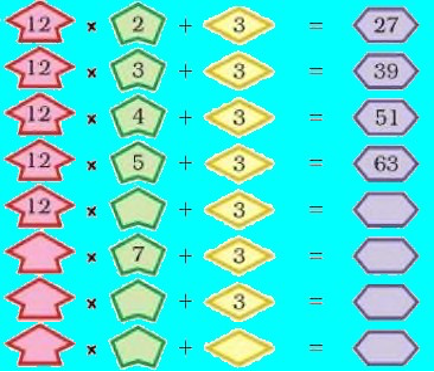
Answer:
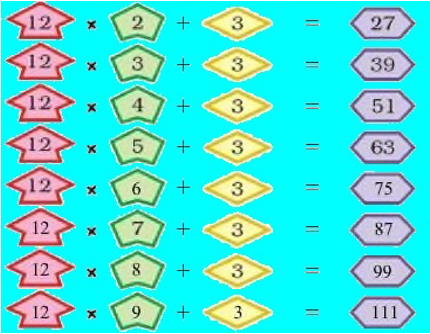
Difference between 39 − 27 = 12 Difference between 51 − 39 = 12
Difference between 63 − 51 = 12 Difference between 75 − 63 = 12
Difference between 87 − 75 = 12 Difference between 99 − 87 = 12 Thus, the difference is same at all the steps.
Question 2: Look at the numbers below. Look for the pattern. Can you take it forward?
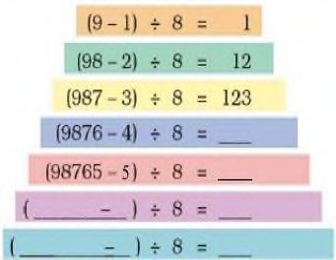
Answer:

Page No 109:
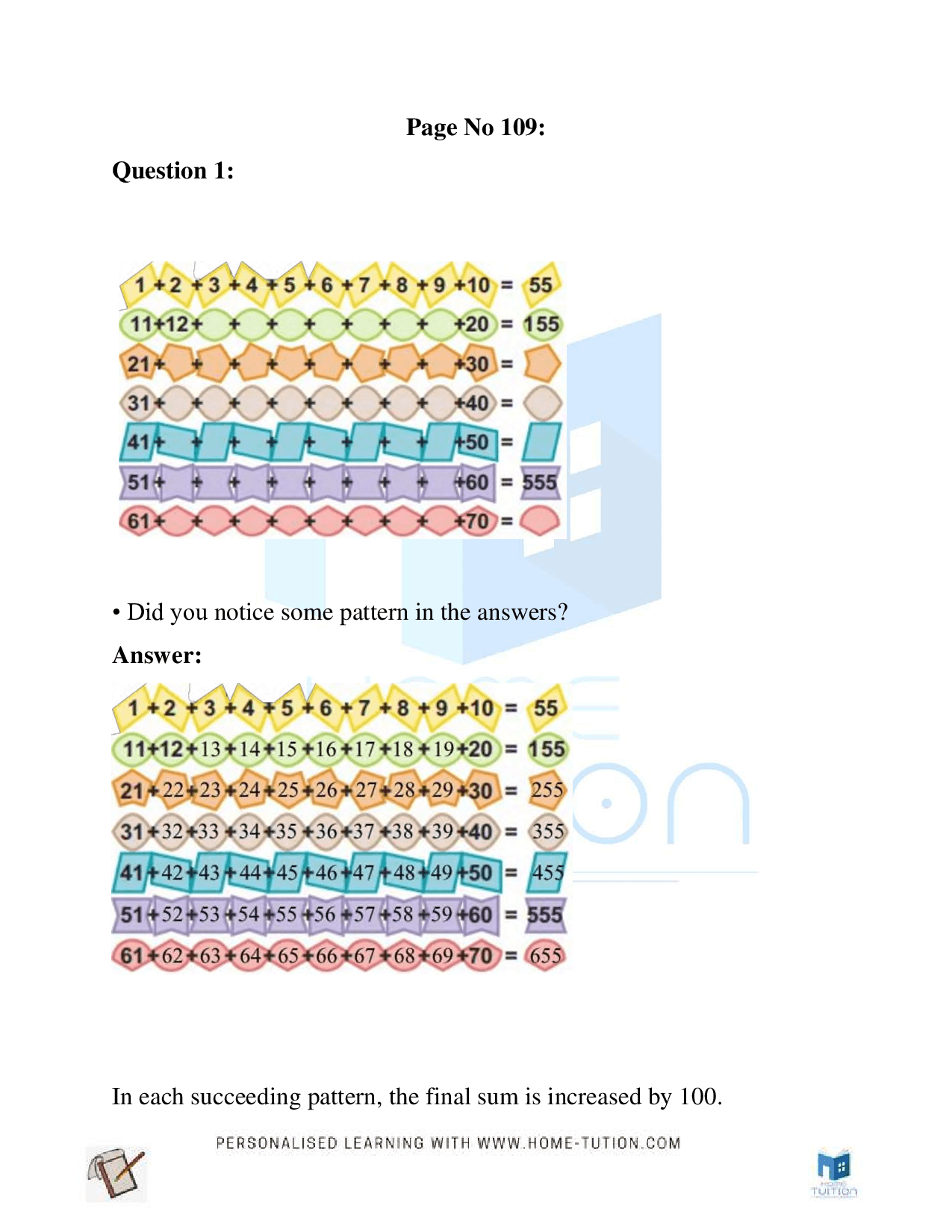
Question 1:
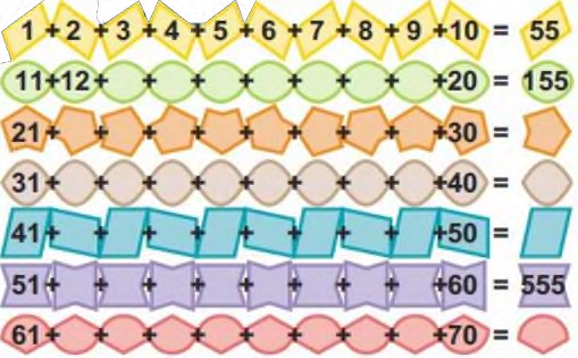
Did you notice some pattern in the answers?
Answer:

In each succeeding pattern, the final sum is increased by 100.
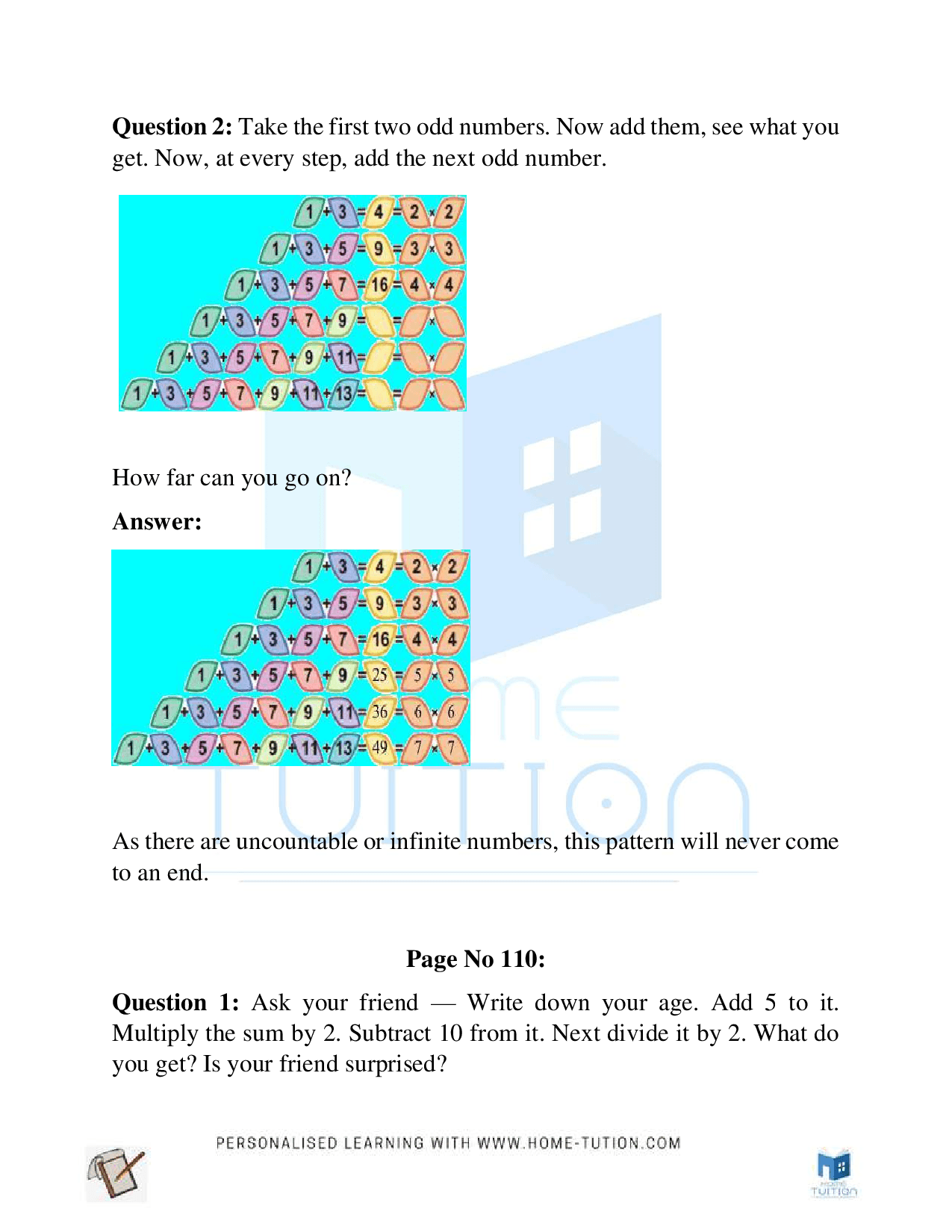
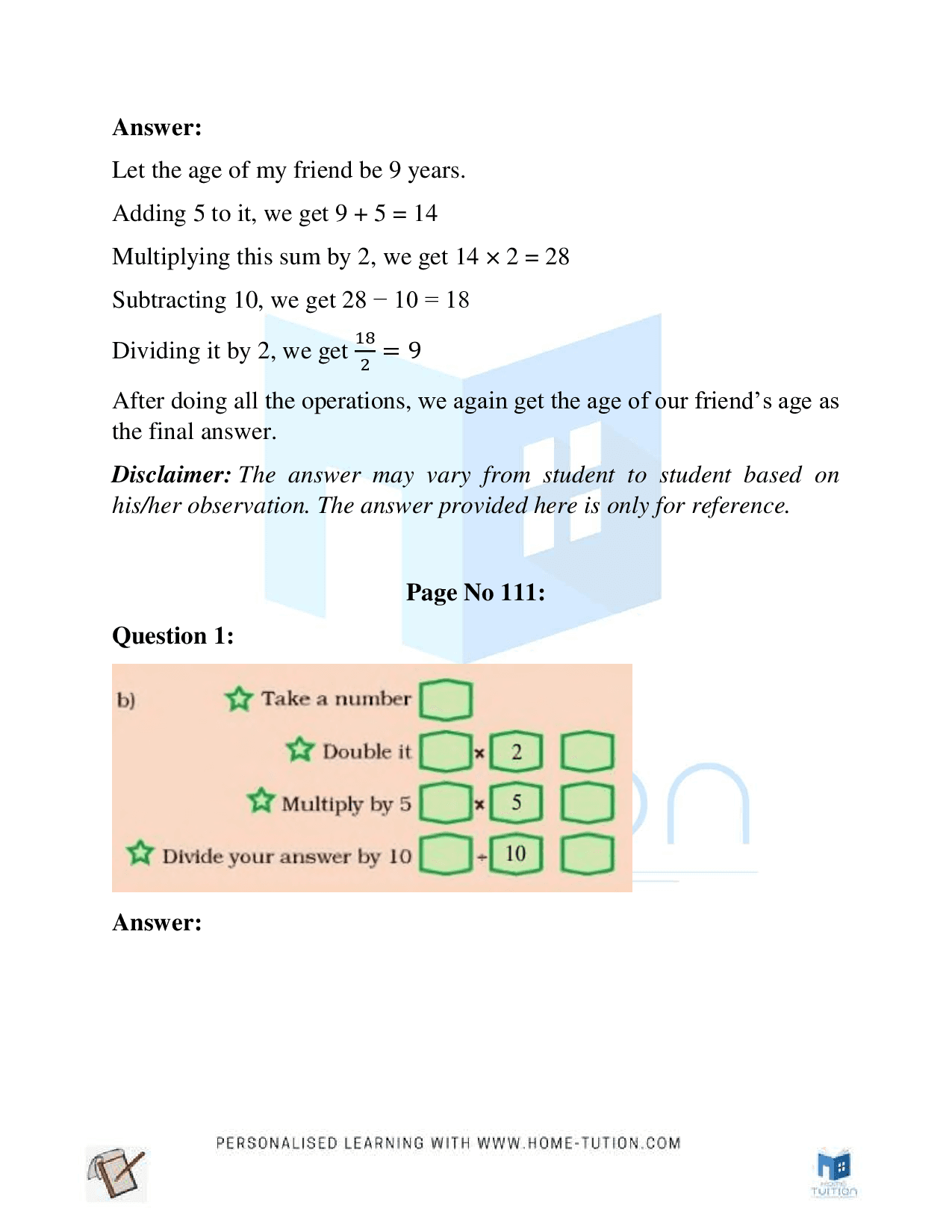
Question 2: Take the first two odd numbers. Now add them, see what you get. Now, at every step, add the next odd number.

How far can you go on?
Answer:

As there are uncountable or infinite numbers, this pattern will never come to an end.
Page No 110:
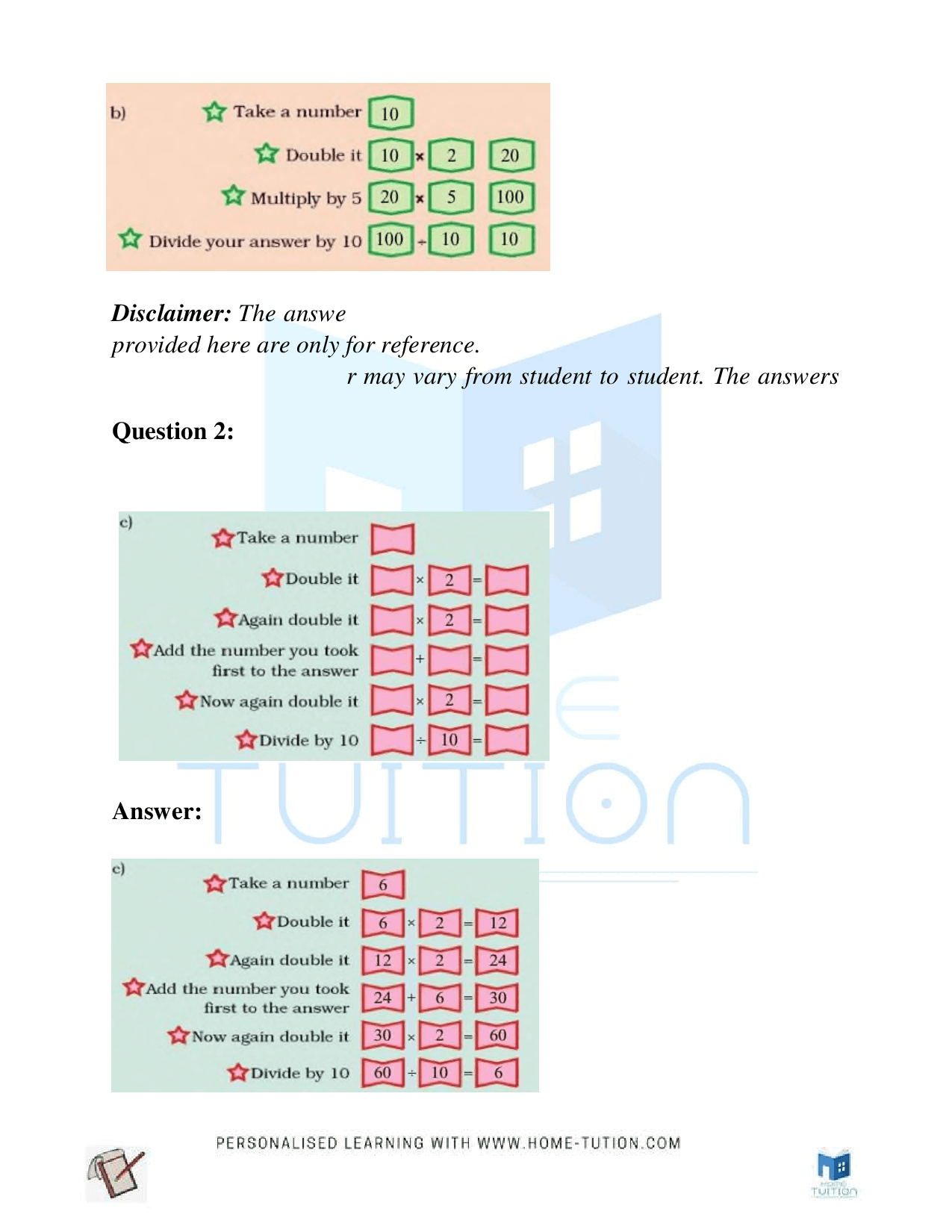
Question 1: Ask your friend — Write down your age. Add 5 to it. Multiply the sum by 2. Subtract 10 from it. Next divide it by 2. What do you get? Is your friend surprised?
Answer:
Let the age of my friend be 9 years. Adding 5 to it, we get 9 + 5 = 14
Multiplying this sum by 2, we get 14 × 2 = 28
Subtracting 10, we get 28 − 10 = 18
Dividing it by 2, we get 18 / 2= 9
After doing all the operations, we again get the age of our friend’s age as the final answer.
Disclaimer: The answer may vary from student to student based on his/her observation. The answer provided here is only for reference.
Page No 111:
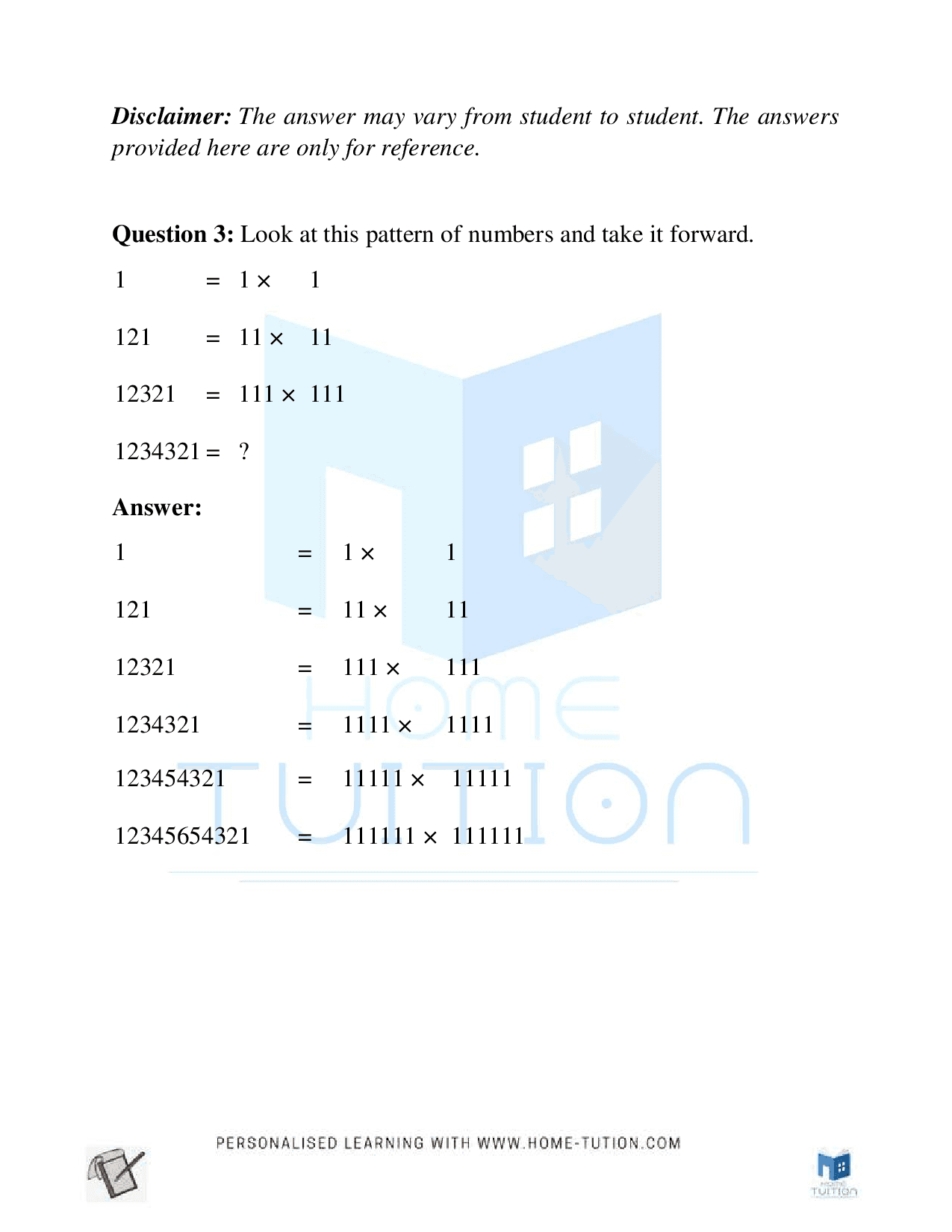
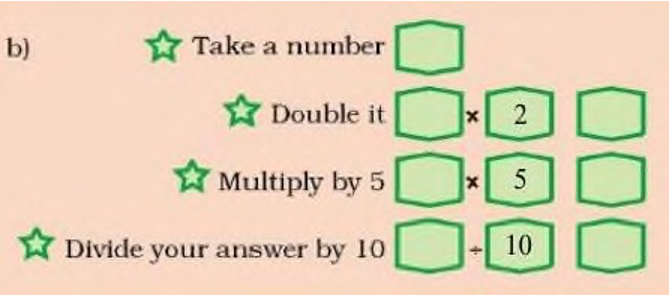
Question 1:
Answer:
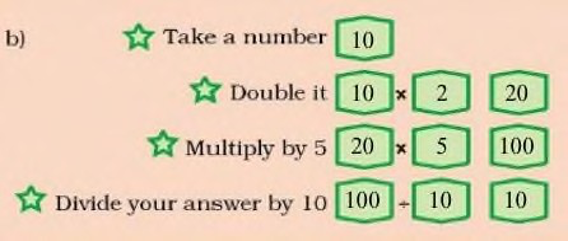
Disclaimer: The answer provided here are only for reference. r may vary from student to student. The answers
Question 2:
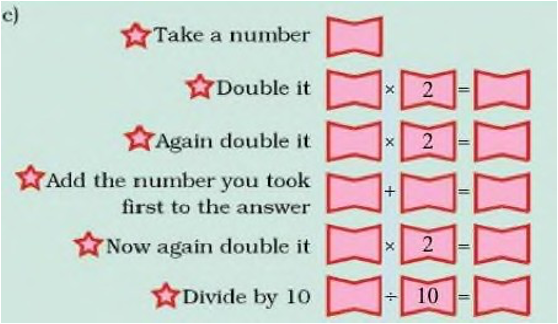
Answer:
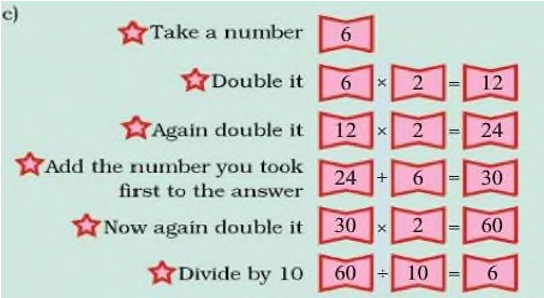
Disclaimer: The answer may vary from student to student. The answers provided here are only for reference.
Question 3: Look at this pattern of numbers and take it forward.
|
1 |
= |
1 × |
1 |
|
121 |
= |
11 × |
11 |
|
12321 |
= |
111 × |
111 |
|
1234321 |
= |
? |
Answer:
|
1 |
= |
1 × |
1 |
|
121 |
= |
11 × |
11 |
|
12321 |
= |
111 × |
111 |
|
1234321 |
= |
1111 × |
1111 |
|
123454321 |
= |
11111 × 11111 |
|
|
12345654321 |
= |
111111 × 111111 |