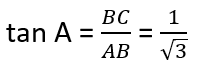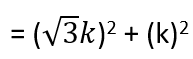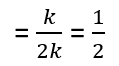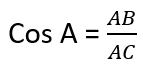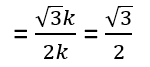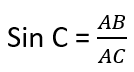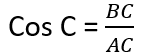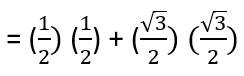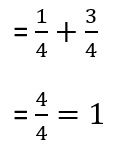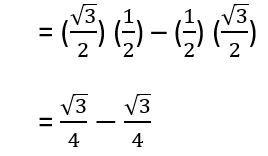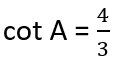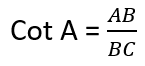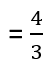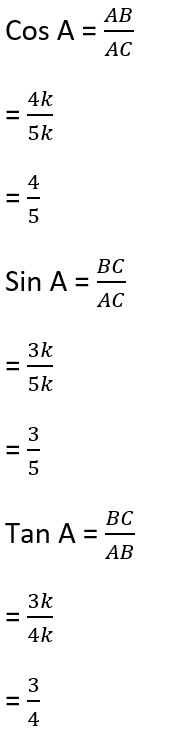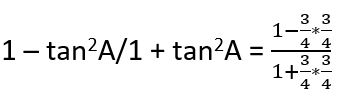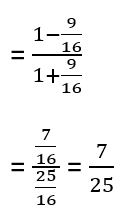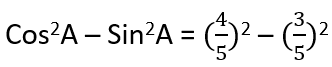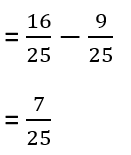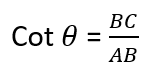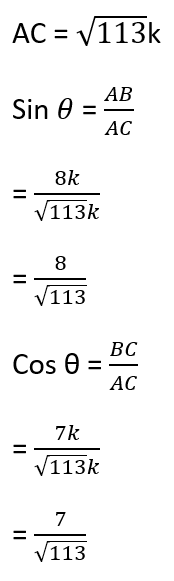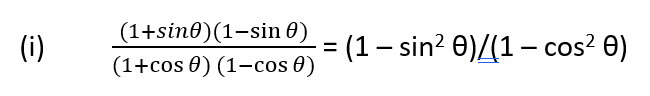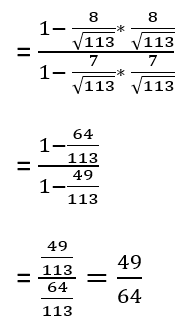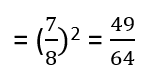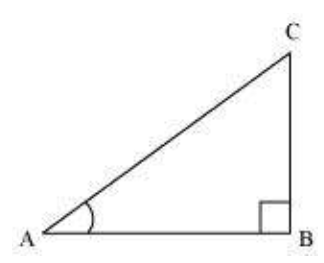
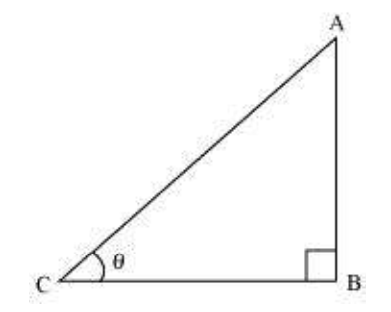
(i) Consider a ΔABC, right-angled at B

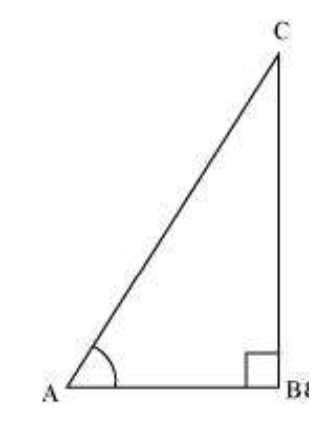
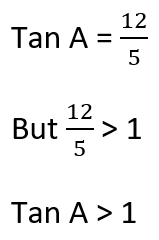
So, Tan A < 1 is not always true

Hence, the given statement is false

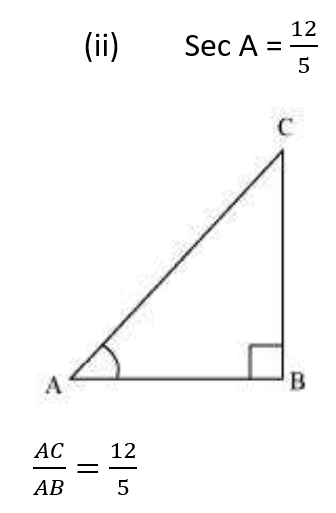
Let AC be 12k, AB will be 5k, where k is a positive integer

Applying Pythagoras theorem in ΔABC, we obtain

AC² = AB² + BC²

(12k)² = (5k)² + BC²

144k² = 25k² + BC²

BC² = 119k²

BC = 10.9k

It can be observed that for given two sides AC = 12k and AB = 5k,

BC should be such that,

AC - AB < BC < AC + AB

12k - 5k < BC < 12k + 5k

7k < BC < 17 k

However, BC = 10.9k. Clearly, such a triangle is possible and hence, such value of sec A is possible Hence, the given statement is true

(iii)Abbreviation used for cosecant of angle A is cosec A. And Cos A is the abbreviation used for cosine of angle A Hence, the given statement is false

(iv)Cot A is not the product of cot and A. It is the cotangent of ∠A

Hence, the given statement is false

(v)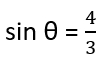             

In a right-angled triangle, hypotenuse is always greater than the remaining two sides. Therefore, such value of sin θ is not possible

Hence, the given statement is false

Given that, PR + QR = 25

PQ = 5

Let PR be x

Therefore,

QR = 25 - x

Applying Pythagoras theorem in ΔPQR, we obtain

PR² = PQ² + QR²

x² = (5)² + (25 - x)²

x² = 25 + 625 + x² - 50x

50x = 650

x = 13

Therefore,

PR = 13 cm

QR = (25 - 13) cm = 12 cm

If BC is k, then AB will be, where k is a positive integer

In ΔABC,

AC² = AB² + BC²

= 3k² + k² = 4k²

AC = 2k

(i) sin A cos C + cos A sin C

(ii) cos A cos C - sin A sin C

= 0

It is given that 3cot A = 4

Or,

Consider a right triangle ABC, right-angled at point B

If AB is 4k, then BC will be 3k, where k is a positive integer

In ΔABC,

(AC)² = (AB)² + (BC)²

= (4k)² + (3k)²

= 16k² + 9k²

= 25k²

AC = 5k

Therefore,

1 – tan²A/1 + tan²A = Cos²A – Sin²A

Let us consider a right triangle ABC, right-angled at point B

=7/8  If BC is 7k, then AB will be 8k, where k is a positive integer.

Applying Pythagoras theorem in ΔABC, we obtain

AC² = AB² + BC²

= (8k)² + (7k)²

= 64k² + 49k²

= 113k²

(ii) Cot² θ = (cot θ)²